8.3.2 Slope of Parallel & Perpendicular Lines
Understand and apply the relationships between the slopes of parallel lines and between the slopes of perpendicular lines. Dynamic graphing software may be used to examine these relationships.
Analyze polygons on a coordinate system by determining the slopes of their sides.
For example: Given the coordinates of four points, determine whether the corresponding quadrilateral is a parallelogram.
Given a line on a coordinate system and the coordinates of a point not on the line, find lines through that point that are parallel and perpendicular to the given line, symbolically and graphically.
Overview
Standard 8.3.2 Essential Understandings/Ideas:
Student learning is focused on identifying parallel and perpendicular lines by comparing the slopes of each line. Students have developed the understanding that parallel lines never cross and perpendicular lines intersect, creating 90-degree angles usually as it pertains to polygons, most likely parallelograms and rectangles. In this standard, students connect this basic understanding to their knowledge of slope. Students will find the slopes of the side of polygons on a coordinate grid to determine the relationship and identify the shape. It is essential for students to move from identifying parallel and perpendicular based on what it “looks like” to identifying parallel and perpendicular by finding the slopes of the sides lengths of the polygon and proving their relationship. It is essential that students know that slopes of parallel lines are the same and perpendicular lines have slopes that are negative reciprocals. These geometric images are useful aids to help student’s reason algebraically about linear equations. After understanding this geometric relationship, students can apply this understanding of slope to write the equations of lines and graph lines that are parallel and perpendicular.
Benchmark Group A
- 8.3.2.1 Understand and apply the relationships between the slopes of parallel lines and between the slopes of perpendicular lines. Dynamic graphing software may be used to examine the relationships between lines and their equations.
- 8.3.2.2 Analyze polygons on a coordinate system by determining the slopes of their sides.
- For example: Given the coordinates of four points, determine whether the corresponding quadrilateral is a parallelogram.
- 8.3.2.3 Given a line on a coordinate system and the coordinates of a point not on the line, find lines through that point that are parallel and perpendicular to the given line, symbolically and graphically.
What students should know and be able to do [at a mastery level] related to these benchmarks.
- Use coordinates of vertices and equations to identify and describe parallel and perpendicular lines
- Find the slope of any line given two coordinate pairs
- Compare slopes of two lines. Identify slopes that are the same for parallel lines and slopes that are negative reciprocals as perpendicular lines.
- Given the coordinates of a shape on a coordinate grid, students will be able to identify the shapes by comparing the slopes of the lines that create the shape.
- Write an equation of the line that passes through a given point that is parallel or perpendicular to a given line.
- Transform an equation from any given form into slope intercept form.
- Write an equation of a line, given a point and the slope.
Work from previous grades that supports this new learning includes:
- Students know how to calculate the slope of a line given a graph, table, equation, or points on the line.
- Write a linear equation given two points.
- Understand the basic characteristics of parallel and perpendicular lines.
- Students know the properties of polygons
- Students know how to recognize ordered pairs given on a coordinate grid.
- Students know how to translate between standard form, slope-intercept form and point-slope form. (learned in 8th grade PRIOR to this benchmark)
NCTM Standards:
- NCTM 6-8 geometry
Specify locations and describe spatial relationships using coordinate geometry and other representational systems.
Expectations: In grades 6–8 all students should—
- use coordinate geometry to represent and examine the properties of geometric shapes.
- use coordinate geometry to examine special geometric shapes, such as regular polygons or those with pairs of parallel or perpendicular sides.
CCSS:
G-GPE (High School Geometry)
Use coordinates to prove simple geometric theorems algebraically
#5: Prove the slope criteria for parallel and perpendicular lines and use them to solve geometric problems (e.g. find the equation of the line parallel or perpendicular to a given line that passes through a given point)
Misconceptions
- Students may sometimes look at two lines and assume they are either parallel or perpendicular without checking slopes. To be sure, students should calculate the slope.
- Students have a difficulty understanding negative reciprocal.
- Students don’t pay attention to the slope as negative or positive. This makes it difficult for them to identify slopes as being the same or negative reciprocals.
- Line segments that are different in length CAN have the same slope. If they just do the rise/run, students get stuck if the fractions are not the same. For example ⅔ and 4/6 are the same slope or -1/2 and 4/2 are negative reciprocals
- Students sometimes believe line segments are either parallel or perpendicular and nothing else.
- When asked to find an equation that is parallel to a given equation, sometimes students will give an equation that has the same slope, but also the same y-intercept. This is the same line, NOT a parallel line.
- When asked to find an equation that is perpendicular to a given equation, sometimes students believe that the perpendicular line MUST have the same y-intercept. This is not necessary in order for two lines to be perpendicular.
- Students think a slope of 0/2 and slope of 0/-4 are neither parallel nor perpendicular.
- When given a slope that is zero (0/2 for example) and asked to find a perpendicular line, students find the opposite reciprocal of -2/0. Students often don’t make the connection that this slope is undefined.
Vignette
Teacher: Name these two quadrilaterals.
Graph A Graph B
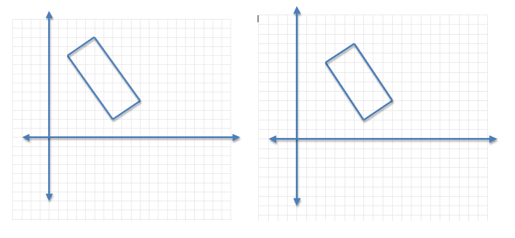
Student: They are both rectangles.
Teacher: How do you know?
Student: Well, it just looks like one. You know the opposite sides are the same length.
Teacher: OK, what else do you know about rectangles?
Student: Opposite sides are parallel and they have four right angles.
Teacher: We need to move beyond that it “looks like” a rectangle and prove that it has the characteristics you described. We are going to focus on the opposite sides being parallel and the adjacent sides being perpendicular or creating a right angle. Let’s look at these four sets of lines.
Graph W Graph X Graph Y Graph Z
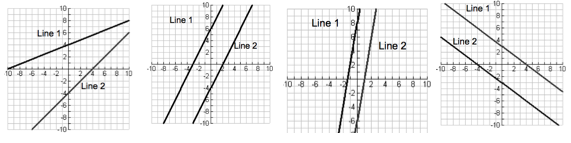
Teacher: What do you notice about the sets of lines?
Student 1: Graphs X, Y, Z are all parallel lines and Graph W is not.
Teacher: How do you know?
Student: It looks like it.
Teacher: Let’s explore the slopes of the lines. Find the slopes of each line. What do you notice?
Student: The lines that look parallel have the same slope. So if two lines have the same slope they must be parallel!
Teacher: Let’s go back to our quadrilaterals. You told me that opposite sides are parallel. So let’s check it out. Find the slopes of the opposite sides of each quadrilateral.
Student: In graph A, the slopes of the “short” sides are 2/3 and 2/3 so they are parallel to each other. The “long” sides have slopes of 7/-5 and 7/-5 so they are parallel to each other too.
In graph B, the “short” sides have a slope of 2/3 and 2/3 and the “long” sides have a slope of 6/-4 and 6/-4 so the opposite sides of this quadrilateral are parallel. Can we say it’s a rectangle now?
Teacher: You mentioned that a rectangle also has 90-degree angles. So we have to prove that the adjacent sides are perpendicular. Let’s look at another set of lines on a coordinate graph. Which of these graphs “look” perpendicular
Graph C Graph D Graph E Graph F

Student: Well, it looks like the first three, graphs C, D, and E are perpendicular but I’m not totally sure.
Teacher: Let’s look at the relationship between the slopes again. Find the slopes of each set of lines. What do you notice?
Student:
|
|
Slope Line 1 |
Slope Line 2 |
|
Graph C |
3/1 |
-1/3 |
|
Graph D |
-4/1 |
1/4 |
|
Graph E |
2/1 |
-1/2 |
|
Graph F |
1/3 |
-2 |
Student: So it looks like one of the lines has a positive slope and one has a negative slope. This is true for all of them, though Graph F doesn’t seem like it’s perpendicular. It also looks like Line 1 and Line 2 are also “flips” of each other.
Teacher: What do you mean by “flips”?
Student: I think it’s called reciprocal. The fraction is flipped and reciprocals multiply to be one.
Teacher: Slopes of perpendicular lines must have both of these qualities. The slopes need to be reciprocals and negatives of each other. Let’s go back to our quadrilaterals. We need adjacent sides that are perpendicular in order to the quadrilateral to be a rectangle. We can check the slopes of the adjacent sides to see if they are negative reciprocals.
Student: We already know the slopes because we found them to check parallel lines. In Graph A, the adjacent sides have slopes of 2/3 and -7/5. They are negative, but they are not reciprocals so they can’t be perpendicular. It really looks like a rectangle but it can’t be because the lines don’t form a right angle. Let’s check Graph B. The slopes of the adjacent sides are 2/3 and -6/4. Again, they are negatives, but they don’t look like reciprocals.
Teacher: Is there another way to write -6/4?
Student: Yes. I can write an equivalent fraction of -3/2. Oh, that is a reciprocal. So yes, Graph B is a rectangle. Even though they both look like rectangles, they aren’t. I see why I have to check the relationship between slopes in order to make sure it fits the characteristics of the shape.
Additional Instructional Resources
Attachment A, Characteristics of Quadrilaterals
Attachment B, The Final Analysis
Attachment C, Coordinate Map Worksheet
Attachment D, Counting Techniques for Coordinate Planes
Attachment E, Identifying Quadrilaterals worksheet
Attachment F, Creating Quadrilaterals Worksheet
Attachment G, Answers to Worksheets
A video explaining parallel and perpendicular lines with examples such as finding slopes given two points and determining if the lines would be parallel/perpendicular or given a line and a point write the perpendicular equation. Steps for solving are shown.
Real life parallel and perpendicular A YouTube video that is an introduction to parallel and perpendicular lines and where one can see them in everyday life. The instruction part of the video is okay (be careful with “inverse reciprocal”), but the real life example pictures of parallel and perpendicular lines are good.
Geogebra lesson A geogebra applet that allows students to create parallel and perpendicular lines in a coordinate grid, calculate the slopes, and look for a relationship between the slopes.
Homework video tutor on parallel and perpendicular lines
Geometer’s Sketchpad activities on investigating slopes of parallel and perpendicular lines and then classifying polygons with slope of lines
A website that shows graphs of parallel and perpendicular lines and asks users to predict whether two lines are parallel or perpendicular. At the bottom of the page is a link to a supplemental worksheet.
A practice worksheet on finding the parallel/perpendicular equations given a point and another equation. Also practice on graphing two equations and determining if they are parallel/perpendicular/neither.
Another practice worksheet on finding the parallel/perpendicular equations.
Resources
Teacher Notes
- There are two ways to think about slopes of perpendicular lines: 1) The slopes are negative reciprocals of each other. 2) Their product equals -1.
- Expect and encourage students to prove their observation of parallel or perpendicular by finding the slope of the line.
- Provide students with a variety of equations in different forms (standard, slope intercept, point slope). Also, variations of slope ½ and 2/4 (parallel) or -3 and 2/6 (perpendicular).
- Be careful using vocabulary. Students interpret words in different ways. For example the word opposite: teacher is meaning opposite sign students may interpret as ¾ and 4/3 as “opposite” because the numbers are flipped.
- Give students examples when the slope is JUST negative -3/2 and 3/2 as well as slopes that are JUST reciprocals ¾ and 4/3 so that students an know that BOTH need to be true in order to have perpendicular lines.
- Expect students to check the slope in the equation to what they draw on the graph. Many times they don’t pay attention to positive/negative slope and even though the slope is positive, they line is decreasing or vice versa.
- Teachers need to stress that questions regarding parallel and perpendicular lines are not asking if two lines “look” parallel or perpendicular, the questions are asking if the “are” parallel or perpendicular which requires students to do the algebra. In the example below, the image “looks like” a rectangle. However, the slopes of the adjacent sides are not negative reciprocals, therefore not perpendicular. So this shape cannot be a rectangle.
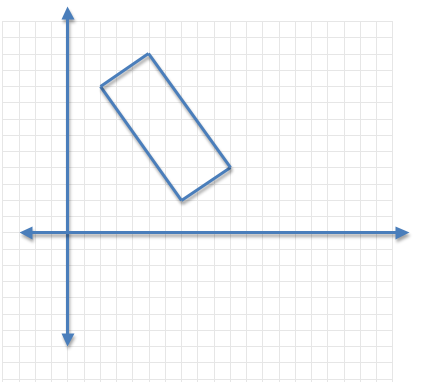
- Teachers need to stress the two steps needed to find perpendicular slope - 1) find the reciprocal and 2) change the sign. Most times if one of the steps is forgotten it is changing the sign of the slope. Teachers could point out that for two lines to be perpendicular one needs to be increasing (positive slope) and the other needs to be decreasing (negative slope). Therefore perpendicular slopes can NEVER have the same sign. Students should make the connection of opposite signs for the perpendicular slopes by referring back to the graph of the perpendicular lines.
- In benchmark 8.3.2.3 students are required to write the equation of a line given a point and a slope. In order to interpret the meaning of the line, the equation is best written in slope-intercept form. Students may use point-slope form, substitution of slope and coordinate point into slope-intercept form and solve for the y-intercept, or use a table of values to write their final equation in slope-intercept form.
- Be intentional about discussing lines that are vertical and horizontal. Discuss with students what it means to have a slope of 0 and a slope that is undefined. Encourage students to plot the points and draw the line so they make a connection between the line and the result of plugging coordinate pairs into the slope formula.
- It is important for students to have access to grid/graph paper when working problems dealing with these benchmarks.
Reciprocal - Two numbers whose product is 1
For example:
6 and 1/6 are reciprocals because 6 x 1/6 = 1.
m and 1/m are reciprocals because m x 1/m = 1.
Negative reciprocal - Two numbers whose product is -1.
For example:
6 and -1/6 are negative reciprocals because 6 x -1/6 = -1.
-m and 1/m are negative reciprocals because -m x 1/m = -1.
Reflection – Critical Questions regarding the teaching and learning of these benchmarks
- What should I change from my current instruction to meet these learning goals?
- Do the students know multiple methods for writing a linear equation given the point and a slope?
- How will I engage students in these lessons?
- How can I teach the concept of parallel and perpendicular to reach all different learning styles?
- What questions can I use as a “ticket out the door” to see if they have understood the days concept?
- How can I tell if they are engaged?
- Do the tasks I’ve designed connect to underlying concepts or focus on memorization
Materials – suggest articles and books
Detective Slope-An Investigation of the Slopes of Lines and Shapes. (2009, January 15). National Security Agency Central Security Services. Retrieved June 13, 2011
Focus in grade 8: teaching with curriculum focal points.. (2010). Reston, VA: National Council of Teachers of Mathematics.
Frayer Model. (n.d.). West Virginia Department of Education. Retrieved June 13, 2011 from http://wvde.state.wv.us/strategybank/FrayerModel.html
Kipfer, J. (n.d.). Parallel and Perpendicular Lines - GeoGebra Dynamic worksheet. GeoGebra. Retrieved June 13, 2011
Lines, Lines, Lines!!! Parallel and Perpendicular Lines. (n.d.). Teacher Bulletin. Retrieved June 13, 2011 from http://www.teacherbulletin.org/TB_VOL10/PDF_files/lines/08%20Lines,%20L…
Parallel and Perpendicular Lines. (n.d.). Clacksmas Middle College. Retrieved June 13, 2011
Parallel lines have the same slope while the slope of perpendicular lines are negative reciprocals and... . (n.d.). Interactive Math Activities, Demonstrations, Lessons with definitions and examples, worksheets, Interactive Activities and other Resources. Retrieved June 13, 2011 from http://www.mathwarehouse.com/algebra/linear_equation/parallel-perpendic…
Pearson Prentice Hall: Web Codes. (n.d.). Prentice Hall Bridge page. Retrieved June 13, 2011
Principles and standards for school mathematics. (2000). Reston, VA: National Council of Teachers of Mathematics.
Quadrilateral Explorations - Grade Nine. (n.d.). Ohio Department of Education. Retrieved June 13, 2011
Regents Exam Quetions G.G.64: Parallel and Perpendicular Lines. (n.d.). Jefferson Math Project. Retrieved June 13, 2011
Assessment
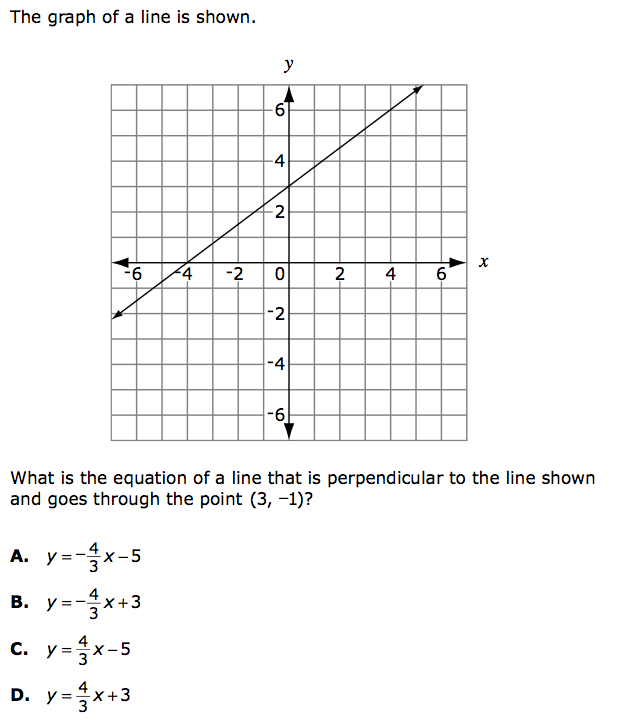
Correct answer: B
From MN MCA III Grade 8 item sampler

Correct answer: A
From MN MCA III Grade 8 item sampler
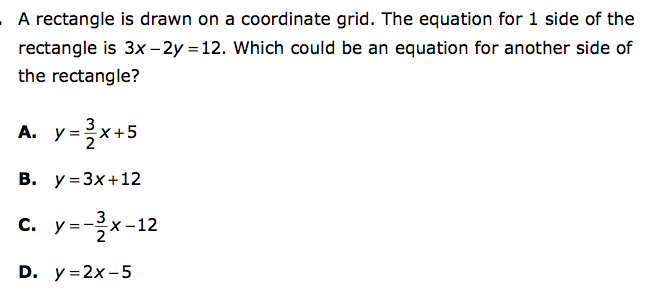
Correct answer: A
From MN MCA III Grade 8 item sampler
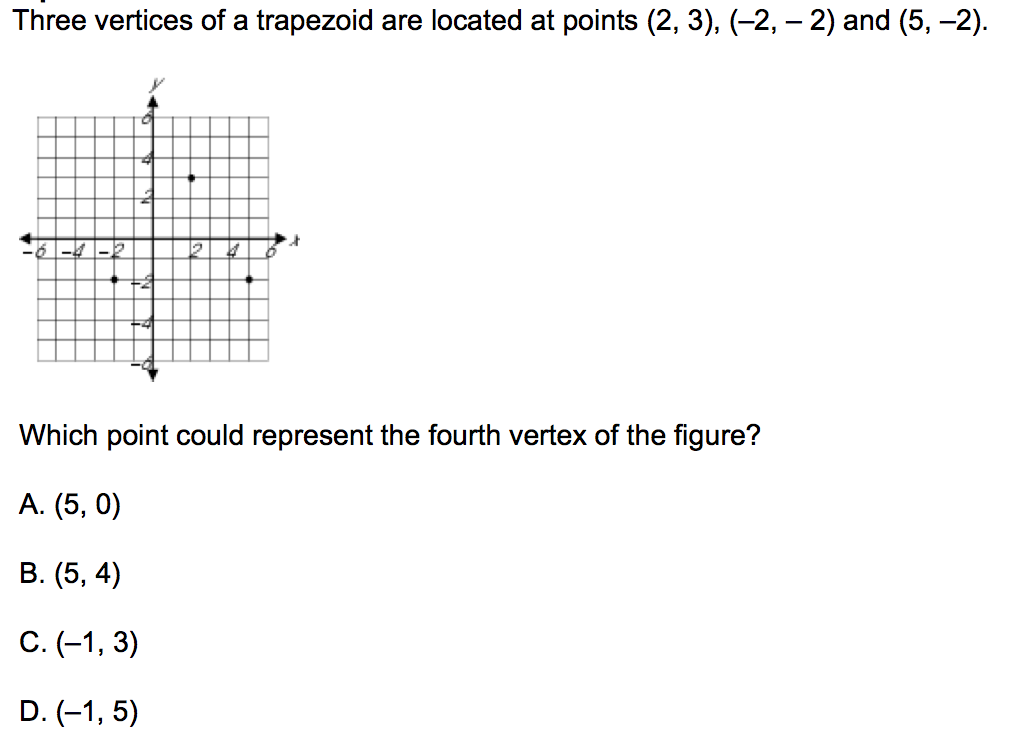
Correct answer: C
What quadrilateral is formed by the following four equations?
y = -x + 2
y = x + 4
x + y = 8
-2x + 2y = -12
Correct answer: square
Which lines are perpendicular to y = 1/2x + 3?
y = 2x +3 2x + y = -1 y = -½x + 4 y = -½ x - ⅓ y = -2x - 5 2x - y = 6
Correct Answer:
2x + y = -1
y = -2x – 5
Which are the coordinates of the vertices of a parallelogram?
A (-3, 2), (-1, 3), (2, 2), (0, 1)
B (4, 1), (3, 5), (0, -2), (3, 3)
C (-2, 1), (-3, 2), (-4, 3), (1, 1)
D (0, 0), (1, 2), (6, 2), (4, 0)
Correct answer: A
4 multiple choice questions from regents prep website Parallel and Perpendicular Lines: Find the equation of a line, given a point on the line and the equation of a line perpendicular to the given line
5 multiple choice questions on Parallel and Perpendicular Lines: Find the slope of a perpendicular line, given the equation of a line
Differentiation
Lines, Lines, Lines! parallel & perpendicular lines lesson plan - good step by step (reteach/sped) instructions. This may also be good as a parent resource.
This is a basic maybe good intro to parallel and perpendicular lines.
Some students may find they can best express the concept of parallel lines by counting the slope “up and over.” For students who are still learning how to use formulas, this strategy is acceptable. The same procedure can be used to show congruent segments, without actually “plugging into” the distance formula.
Students having difficulty identifying quadrilaterals which are obliquely oriented (those not oriented with horizontal or vertical sides) may find it helpful to draw on a transparency and rotate the transparency against another with the coordinate axes.
For students who are developing understanding, provide a formula sheet for the post assessment to find slope, distance and reduce the number of exercises and allow counting techniques with appropriate support.
Use geometric software to provide practice and a different media for addressing a variety of learning styles.
(from Ohio website)
Have students who confuse the terms parallel and perpendicular make vocabulary cards and use them to find examples in letters and mathematical symbols. For example, the plus sign has perpendicular lines and the equal sign has parallel lines.
Use sentence frames to help with their vocabulary:
“The difference between parallel and perpendicular lines is that parallel lines ____________________________ and perpendicular lines ____________________________”.
“The slope of a line parallel to this line is ____________________________ perpendicular to this line is ____________________________”.
Have students practice speaking in English by working in partners or in small groups as they practice how to do a particular type of math problem. Encourage them to use math phrases as much as possible as they explain the process aloud. Ask students to repeat a problem or process back to you after you explain it. Ask questions about the problem or the lesson to ensure all of the students understand what you have taught.
Have students make a Frayer Model for parallel and perpendicular lines. Or have them make one for each polygon and the characteristics of each.
For students with advanced understanding, give guidelines for a quadrilateral and have students create the quadrilateral. For example, the quadrilateral has an area of at least 16 square units, one set of parallel sides, is rotated 30 degrees off the axis and is contained in two quadrants. (taken from this site)
Give equations of the sides of the polygons and have students find points of intersection before using the distance formula to find the lengths of sides. This would challenge students to find the coordinates of each vertex before using the formulas in this lesson.
Use transparencies or graphing technology to explore transformations of the quadrilaterals by rotating, translating, or reflecting them and checking which properties stay the same.
Use local maps to plan trips, analyzing what kinds of polygons the round trips would form and using the coordinates to compute distances for the legs of the trip.
Parents/Admin
|
Students Are: (descriptive list) |
Teachers Are: (descriptive list) |
|
Students are graphing 3 equations given to them (all that have the same slope) and looking for similarities. They are then asked to find another equation that would be like the first three. |
giving students opportunities to compare a variety of lines on a graph and the equation
|
|
Students are graphing 2 equations given to them (that have negative reciprocal slopes) and are looking for similarities. They are then asked to come up with another 2 equations that would fit the pattern of perpendicular as well. |
asking students questions about what they notice for all the lines that are parallel and what do they notice for all the lines that are perpendicular. What patterns do you notice in the equations of the pairs of parallel lines? What patterns do you notice in the equations of pairs of perpendicular lines? |
|
Comparing slopes of side lengths of shapes, noticing what parallel lines have in common and what perpendicular lines have in common. |
FIRST giving students an opportunity to look at the slopes of the sides of shapes that they know have parallel and perpendicular lines, THEN transferring that information to the slopes of two lines |
|
Using geometric software to explore the relationships between side lengths of polygons and lines that are parallel/perpendicular. |
using geometric software to provide practice on comparing parallel and perpendicular lines. |
|
graphing pairs of equations that are parallel graphing pairs of equations that are perpendicular graphing pairs of equations that are neither |
providing students with a variety of shapes that due to scale do not look like they have parallel or perpendicular lines, pushing students to really examine slope. |
|
|
providing a variety of forms of equations as the initial equation in the questions regarding benchmark 8.3.2.3 |
Choose Algebra IA and then parallel and perpendicular lines on the right. Has examples and practice questions regarding the relationship between the slopes of parallel and perpendicular lines and writing equations of lines that are parallel/perpendicular to a given line through a given point.
Linear functions: slopes of parallel and perpendicular lines practice problems with explanations if wrong. IXL is a resource that is free initially, but after a certain amount of use in a day becomes a site that needs to be purchased.
Video on how to find the equation of a line perpendicular to a given line through a given point. This video uses substitution into y=mx+b as a method for finding the equation of a line given a point and a slope
Video on finding the equation of lines parallel/perpendicular to a given line. This video uses point-slope form to find the equations.
