4.1.1C Division
Use strategies and algorithms based on knowledge of place value, equality and properties of operations to divide multi-digit whole numbers by one- or two-digit numbers. Strategies may include mental strategies, partial quotients, the commutative, associative, and distributive properties and repeated subtraction.
For example: A group of 324 students is going to a museum in 6 buses. If each bus has the same number of students, how many students will be on each bus?
Overview
Fourth graders continue work with multiplication and division by demonstrating mastery of basic multiplication and division facts. They use basic multiplication facts to support work with the multiplication of multi-digit numbers. In addition, place value understanding supports multiplication by 10, 100 and 1000. Fourth graders solve multi-step problems involving multiplication, addition and subtraction. They divide multi-digit numbers by one- and two-digit numbers in problem solving situations. They solve these division problems using a variety of strategies including
- repeated subtraction
- distributive, associative and commutative properties
- place value understanding
- mental strategies
- partial quotients
Fourth graders use rounding, benchmark numbers and place value to estimate and evaluate the reasonableness of results.
All Standard Benchmarks
4.1.1.1 Demonstrate fluency with multiplication and division facts.
4.1.1.2 Use an understanding of place value to multiply a number by 10, 100, and 1,000
4.1.1.3 Multiply multi-digit numbers, using efficient and generalizable procedures, based on knowledge of place value, including standard algorithms.
4.1.1.4 Estimate products and quotients of multi-digit whole numbers by using rounding, benchmarks and place value to assess the reasonableness of results. For example: 53 × 38 is between 50 × 30 and 60 × 40, or between 1500 and 2400, and 411/73 is between 5 and 6.
4.1.1.5 Solve multi-step real-world and mathematical problems requiring the use of addition, subtraction and multiplication of multi-digit whole numbers. Use various strategies, including the relationship between operations, the use of technology, and the context of the problem to assess the reasonableness of results.
4.1.1.6 Use strategies and algorithms based on knowledge of place value, equality and properties of operations to divide multi-digit whole numbers by one- or two-digit numbers.
Strategies may include mental strategies, partial quotients, the commutative, associative, and distributive properties and repeated subtraction. For example: A group of 324 students is going to a museum in 6 buses. If each bus has the same number of students, how many students will be on each bus?
4.1.1.6 Use strategies and algorithms based on knowledge of place value, equality and properties of operations to divide multi-digit whole numbers by one- or two-digit numbers.
Strategies may include mental strategies, partial quotients, the commutative, associative, and distributive properties and repeated subtraction. For example: A group of 324 students is going to a museum in 6 buses. If each bus has the same number of students, how many students will be on each bus?
What students should know and be able to do [at a mastery level] related to these benchmarks:
- solve both partitive and measurement division problems with dividends containing at most three-digits;
- develop fluency with efficient procedures for dividing whole numbers with dividends containing at most three digits;
- apply their understanding of models for division, place value, properties of operations, and the relationship of division to multiplication as they
- develop, discuss, and use efficient, accurate, and generalizable procedures to find quotients involving multi-digit dividends;
- select and accurately apply appropriate methods to estimate and mentally calculate quotients;
- explain what a remainder means and how to use it when finding a solution to a division problem. These division problems need to be in a context.
Work from previous grades that supports this new learning includes:
- understanding how to solve real world and mathematical problems that include both "how many in each group" and "how many groups" as a means of solving sharing (division) problems;
- using various strategies to represent division facts such as repeated subtraction, equal sharing and forming equal groups;
- recognizing the relationship between multiplication and division.
NCTM Standards
Understand numbers, ways of representing numbers, relationships among numbers, and number systems
Grades 3 - 5 Expectations
- understand the place-value structure of the base-ten number system and be able to represent and compare whole numbers and decimals;
- recognize equivalent representations for the same number and generate them by decomposing and composing numbers;
- develop understanding of fractions as parts of unit wholes, as parts of a collection, as locations on number lines, and as divisions of whole numbers;
- use models, benchmarks, and equivalent forms to judge the size of fractions;
- recognize and generate equivalent forms of commonly used fractions, decimals, and percents;
- explore numbers less than 0 by extending the number line and through familiar applications;
- describe classes of numbers according to characteristics such as the nature of their factors.
Understand meanings of operations and how they relate to one another
Grades 3 - 5 Expectations
- understand various meanings of multiplication and division;
- understand the effects of multiplying and dividing whole numbers;
- identify and use relationships between operations, such as division as the inverse of multiplication, to solve problems;
- understand and use properties of operations, such as the distributivity of multiplication over addition.
Compute fluently and make reasonable estimates
Grade 3 - 5 Expectations
- develop fluency with basic number combinations for multiplication and division and use these combinations to mentally compute related problems, such as 30 x 50;
- develop fluency in adding, subtracting, multiplying, and dividing whole numbers;
- develop and use strategies to estimate the results of whole-number computations and to judge the reasonableness of such results;
- develop and use strategies to estimate computations involving fractions and decimals in situations relevant to students' experience;
- use visual models, benchmarks, and equivalent forms to add and subtract commonly used fractions and decimals;
- select appropriate methods and tools for computing with whole numbers from among mental computation, estimation, calculators, and paper and pencil according to the context and nature of the computation and use the selected method or tool.
Common Core State Standards
- Generalize place value understanding for multi-digit whole numbers.
4.OA.1. Recognize that in a multi-digit whole number, a digit in one place represents ten times what it represents in the place to its right. For example, recognize that 700 ÷ 70 = 10 by applying concepts of place value and division.
4.OA.2. Read and write multi-digit whole numbers using base-ten numerals, number names, and expanded form. Compare two multi-digit numbers based on meanings of the digits in each place, using >, =, and < symbols to record the results of comparisons.
4.OA.3. Use place value understanding to round multi-digit whole numbers to any place.
- Use place value understanding and properties of operations to perform multi-digit arithmetic.
4.OA.4. Fluently add and subtract multi-digit whole numbers using the standard algorithm.
4.OA.5. Multiply a whole number of up to four digits by a one-digit whole number, and multiply two two-digit numbers, using strategies based on place value and the properties of operations. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
4.OA.6. Find whole-number quotients and remainders with up to four-digit dividends and one-digit divisors, using strategies based on place value, the properties of operations, and/or the relationship between multiplication and division. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
- Generalize place value understanding for multi-digit whole numbers.
4.NBT.1. Recognize that in a multi-digit whole number, a digit in one place represents ten times what it represents in the place to its right. For example, recognize that 700 ÷ 70 = 10 by applying concepts of place value and division.
4.NBT.2. Read and write multi-digit whole numbers using base-ten numerals, number names, and expanded form. Compare two multi-digit numbers based on meanings of the digits in each place, using >, =, and < symbols to record the results of comparisons.
4.NBT.3. Use place value understanding to round multi-digit whole numbers to any place.
- Use place value understanding and properties of operations to perform multi-digit arithmetic.
4.NBT.4. Fluently add and subtract multi-digit whole numbers using the standard algorithm.
4.NBT.5. Multiply a whole number of up to four digits by a one-digit whole number, and multiply two two-digit numbers, using strategies based on place value and the properties of operations. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
4.NBT.6. Find whole-number quotients and remainders with up to four-digit dividends and one-digit divisors, using strategies based on place value, the properties of operations, and/or the relationship between multiplication and division. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
Misconceptions
Student Misconceptions and Common Errors
Students may think....
- additively rather than multiplicatively;
- remainders are decimal answers and can be written as such, for example: 58 ÷ 7 = 8.2 (should be 8 r 2 or 8 2/7);
- they can solve multiplication and division problems the same way they would solve addition and subtraction problems;
- there is only one way to solve a problem;
- "key words" indicate which operation to use when solving a story problem;
- "When dividing, get as close to the number (dividend) as you can and however far away from it you are, that is your remainder."
This is a common error and challenging to undo! Students need to realize they cannot go above the dividend (number being divided) even if it gets them "closer". Using manipulatives to make groups is a helpful way to model this. This happens when students are focused on a procedure that does not make sense to them instead of applying the concept of division. For example: 71 ÷ 8 = 9 remainder 1. 9 x 8 = 72 and that is 1 away (should be 8 r 7).
Vignette
In the Classroom
Vignette I:
Students in this fourth grade classroom have recently been exploring ways to divide 3 digit numbers by 1 digit numbers. There has been no formal teaching of "long division", they have simply been asked to use what they know about multiplication and division to solve problems with larger numbers. Two problems have been provided and the directions given were, "Choose one problem to start with. Create a realistic scenario or reason you might be solving this problem and write it down (Benchmark 4.2.2.1). Then, solve the problem . The problems provided were:
804 ÷ 6 and 846 ÷ 5.
The students worked independently, in pairs, and occasionally with a trio to share their ideas about how to solve the problem and check out other people's strategies and thinking. The portion provided below has two children sharing two different strategies for each of the problems. This is done whole group the last portion of class period.
Problem: 804 ÷ 6
Student A: I chose to solve 804 ÷ 6 because I felt like I could start by thinking about it as 800. The story I made up is, "My friends and I go to Grand Slam. We get 804 tokens for the video games. There are 6 of us, how many do we each get?"
There is lots of approval from the audience for this scenario.
Student A: I don't know how to do the division where you put it in the tent, so I started by thinking about how many groups of 6 are there in 80 instead of 800 because that is easier to think about. I know 6 x 10 is 60, right? So, then I keep counting by 6's, 66, 72, 78 and that is as high as I can go without going over 80. I counted 3 more groups of 6 past the 10 so that is 13 groups of 6. That is 6 x 13 = 78. (At this point the student starts writing on the board). But I need to get to 800 so I do 6 x 130 will get me 780.
Board has this written on it:
804 ÷ 6 =
6 x 13 = 78
6 x 130 = 780
Student from audience: I don't get where the 130 comes from-why 130?
Student A: Well, I need to get to 804, but I was just thinking about 80 at first- remember (points to the 80 in the 804). Then I know that the 13 can be multiplied by 10 to get me 130, so then your answer is multiplied by 10 too.
Audience member still looks confused.
Student B raises hand and is called on to help explain: What A is saying is that if you have 6 piles of 13 tokens, you have 78. Right? But if you have 6 piles of 130, now you have 780 total. 13 + 13 + 13 done six times is 78. So 130 + 130 done six times is 780.
Suddenly lots of kids start nodding their heads and seem to be making the connection.
Student A: Yeah, like that. (Student B sits back down) So now I look at how many away from 804 I am. So I subtract 780 from 804 and I get 24. That is really close. Now I just think, 6 times what is close to 24- and it's easy because 6 x 4 is 24! (adds thinking to board).
Board now has this written on it:
804 ÷ 6 =
6 x 13 = 78
6 x 130 = 780
804-780 = 24
6 x 4 = 24
130 + 4= 134
804 ÷ 6 = 134
Student A: 130 tokens from before, plus these four more means we each get 134 which is way more than I thought it would be.
Second child shares an answer to the same problem:
Student C: I did something sort of like that- my brother does this kind of homework and I get part of it. My story is, "I need $804 to go to the two horse camps I want to go to. If I have 6 months to get that much money, how much do I need to get each month?" It took me a long time to come up with a question that made sense and needed division. Mrs. X helped me- so thanks.
Student starts writing on the board and talking:
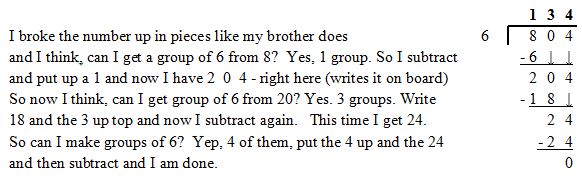
This is all done in a matter of minutes and now the audience has questions:
Student D: I don't get it. Why did you say you can only put 6 into 8 one time and then put the 1 up above the 8?
Student C: Because this way is how you do it.
Student A (from above goes to the board): What I think happens is this is like saying you can put 6 into 800 one-hundred times- see how the one ends up in the hundreds place when C is done? C gets the same answer I did, but is looking at the numbers different.
Student E: The subtracting is sort of confusing, C still just subtracted a 6 from the 804, why 6?
Student C: I multiplied the 6 and the 1 I put up there (points to the 1 in the quotient) and then I put the answer below the 8 because that is the answer to 6 x 1. Then I subtracted the 6 from the 8. See how I moved the zero and four down by the 2? Then I have to find groups of 6 again. I can't take a group of 6 from the 2, so I go to the next place and see that it is zero. I can take groups of 6 from 20.
Student D: That isn't 20, it's 204. I still don't get it.
Student C: In this method, that is how you do it. It is called "long division".
Teacher interjects: This is our first look as a class at long division. It is a popular way to solve division problems. You will understand the steps more once you have had more practice thinking about division in other ways. Let's keep both strategies posted as we see other strategies and then we can look at how they are similar. I think that will help us all understand the different ways of solving division problems.
Problem: 846 ÷ 5.
Student G: I solved 846 ÷ 5. I thought of 846 pennies being traded in for nickels. I sort of did a different problem. I thought of it as 850 ÷ 5 instead since it is so close. Then I thought 5 x ? = 850. I actually did it a like Student A - I thought of 5 x ? = 85 first. I know 5 x 10 is 50 and 5 x 7 is 35 so 5 x 17 = 85.
Board has this written on it:
5 x ? = 850
5 x ?= 85
5 x 10 = 50
5 x 7 = 35
5 x 17 = 85
Student from audience: I don't see how you got to 17- where did the 7 come from?
Student G: I got 50 from 5 x 10, but I need 85. 85 -50 is 35 so then I needed to do 5 x 7 to get 35. I added the 10 from 5 x 10 and the 7 from 5 x 7 because that is how I got to 85.
This is now on the board:
5 x ? = 850
5 x ?= 85
5 x 10 = 50
5 x 7 = 35
5 x 17 = 85
85-50 = 35 (arrow up to 5 x 7 = 35)
10 + 7 = 17 (10 and 7 above circled)
Student from audience: Oh, okay.
Student G: Now I know that 5 x 170 is 850 because it is like what Student A did. (Student G points back to 6 x 13 and 6 x 130 from earlier work and then looks at audience to see if they are following).
Student G: So now I need to figure out what to do because I rounded up to 850 from 846. I had to get help from Mrs. X because I wasn't sure how to undo it. What we figure out is that I added 4 to 846 to get to 850 (counts up on fingers to show audience his thinking) 847, 848, 849, 850- see? (four fingers are up). So, I won't be able to get 170 nickels, I will be four pennies short. I need to get 169 nickels and I will have one penny left. It looks like this:
Writes on board under other work: 846 ÷ 5 = 169 r 1
Student G: Any questions?
Student from audience: I like your strategy but I would be confused on how to undo my rounding when I rounded.
Student G: I was too. We (student and teacher) looked at a smaller problem to help us figure out what to do. If it was just 84 ÷ 5 and I did 85 ÷ 5 instead, I would have one less group of 5 and 4 left over. I just used that to try to figure out what I needed to undo.
Second child shares an answer to the same problem:
Student H: Well, as you all know, I really like to work with expanded notation. So I thought of the problem like this: Writes on board:
800 ÷ 5 =
40 ÷ 5 =
6 ÷ 5 =
First I did what Student A did and thought what times 5 will get me to 80? I know there are two groups of 5 in every group of ten and 80 is 8 groups of 10 so I know it was 16.
Audience snaps in approval.
Student H: But I need to get to 800 so it is 160. So 800 ÷ 5= 160. The next two parts are simple! 40 ÷ 5 = 8 and 6 ÷ 5 = 1 with 1 left over. (This is spoken while the equations below are solved)
Writes on board:
800 ÷ 5= 160
40 ÷ 5 = 8
6 ÷ 5 = 1 R 1
Student H: Now I just add up my answers and I get 169 R 1
Writes on board: 160 + 8 + 1 R1 = 169 R 1.
Responses from audience:
"I really like that way of doing the problem."
"It makes sense to me."
"You are really good at solving problems with expanded notation."
"Does this work for all division problems?"
Teacher is out of time for today's lesson and writes the final response on the board to be considered for tonight's homework.
Vignette II
(Adapted from the 1997 MN Mathematics Framework, chapter 3, pg. 18-23.)
"I have a number sentence I want you to look at and tell me what you think," Ms. Davis began while writing on the board:
"126 / 9 = ?"
Student: "It's a division."
Student: "That's right. The sign tells us that."
Student: "It would help if we knew where it came from."
Ms. Davis: "Why do you say that?"
Student: "Well, when I know where a number sentence comes from I can get a better feel for what I have to do."
There was general agreement from the class here. Mathematics for them had been about situations.
"Who can suggest a situation which would produce this number sentence?" interjected Ms. Davis. (4.2.2.1)
Student: "I can. I have 126 trick or treat candies to share among 9 friends, how many will they each get?"
Student: "But it could be something else."
Ms. Davis: "It could?"
Student: "Yes, it could be, 'I have 126 spare baseball cards to share with my 8 friends.' "
Ms. Davis: "I assume you are included with that?"
Student: "It's the same problem as before."
Student: "I've got one that is different."
Ms. Davis: "Go ahead, let's listen to this new one."
Student: "There are 126 trick or treat candies. If I put 9 in a bag, how many bags can I fill?"
Student: "That is different."
Ms. Davis: "So what should we do now? We seem to have two problems from the same number sentence."
Student: "Make a model!"
Ms. Davis: "Of both? OK, I'll tell you what we can do. Let's split the class into two groups and one half can model one problem and the other half the second one. Then we can come together to discuss what we have done and critique our findings."
The class began working in two groups while Ms. Davis walked around asking questions to redirect groups or to check understanding. The fifth graders were acting out each situation and developing an algorithm as they went along. The algorithm was becoming rather like a mathematical commentary on the action. After a while Ms. Davis called the class together to analyze their results.
"Now, Hallie, you are the spokesperson for your group. Tell us what you did."
Hallie came to the front of the room with a chart and began.
"We didn't have any candies so we used these cubes to represent the candies. We put out 126 and then we chose 9 of us to be the lucky ones who got the candies. First we gave them out, saying 'One for you', and 'One for you', and 'One for you', and so on until they all had one.
"After this there were some more left to share so we again said 'One for you,' and 'One for you,' and so on until they all had another one. We kept on like that until all the candies were given out. Then we counted how many each person had. It was 14. We checked by multiplying 14 by 9. We wrote down what we had done as we shared:"
Hallie wrote the following:
126 81 36
- 9 1 each - 9 1 each -9 1 each
117 72 27
- 9 1 each - 9 1 each - 9 1 each
108 63 18
- 9 1 each - 9 1 each - 9 1 each
99 54 - 9
- 9 1 each - 9 1 each - 9 1 each
90 45 0
- 9 1 each - 9 1 each
81 36
That is a total of 14 each. 14 x 9 = 126
"We decided this was a very long way to do it, but we are sure we got the right answer," said Hallie.
Choua interrupted Hallie's report, saying, "We did the same thing but when we wrote it down, we didn't say '1 each' but '1 bag.' Taking 9 candies from our pile, we called it '1 bag' and then 9 more was another bag, and so on. Our multiplication check is different, but it has the same correct result."
126 81 36
- 9 1 bag - 9 1 bag -9 1 bag
117 72 27
- 9 1 bag - 9 1 bag - 9 1 bag
108 63 18
- 9 1 bag - 9 1 bag - 9 1 bag
99 54 - 9
- 9 1 bag - 9 1 bag - 9 1 bag
90 45 0
- 9 1 bag - 9 1 bag
81 36
That is a total of 14 bags. 9 x 14 = 126
Hallie then continued her report. "We tried sharing in other ways and wrote down each one on a chart."
126 126 126 126
-18 2 each -27 3 each -45 5 each -90 10 each
108 99 81 36
-18 2 each -27 3 each -45 5 each -36 4 each
90 72 36 0
-18 2 each -27 3 each -36 4 each
72 45 0
-18 2 each -27 3 each
54 18
-18 2 each -18 2 each
36 0
-18 2 each
18
-18 2 each
0 Each way gave us 14 candies per person.
This made Choua think about what his group had done. He said, "We did the same thing but we didn't actually share the cubes out into bags as we knew we would get 14. We just tried to improve the way we could write it all down and each time we got a total of 14 bags." Each way gave us 14 bags.
126 126 126 126
-18 2 bags -27 3 bags -45 5 bags -90 10 bags
108 99 81 36
-18 2 bags -27 3 bags -45 5 bags -36 4 bags
90 72 36 0
-18 2 bags -27 3 bags -36 4 bags
72 45 0
-18 2 bags -27 3 bags
54 18
-18 2 bags -18 2 bags
36 0
-18 2 bags
18
-18 2 bags
0 Each way gave us 14 bags.
Ms. Davis said, "What conclusions can we come to for problems like these? Sharing out sets of 10 seems the best to me, the others take so much time."
"Why didn't we do it that way first?"
"I never thought about it. It seemed obvious to share one at a time, but if there are a lot it gets really boring."
"Sets of 10 will not always be the best because when dividing by 9, we have to have at least 90 to share out a set of 10."
"But sets of 10 are very easy to remember. They would be better than sets of 8!"
Ms. Davis, as she wrote: "I think we need to finish these charts. We need to make a number sentence of what both groups found." 126 / 9 = 14 because 14 x 9 = 126
"Audra, please read this for us."
"It says 'One hundred twenty-six divided by 9 equals 14 because fourteen multiplied by nine equals one hundred twenty-six. We had fourteen nine times as a result of our sharing."
"Now, what about your display, Choua? What can your team write?"
"I think it will be the same, everything else has been."
"Not quite. Remember you were filling bags. You know how many candies went into each bag. You were giving to each person. You knew how many people were going to share the candies."
"Oh, I think we would write: 126 / 9 = 14 because 9 x 14 = 126
The multiplication part is different, so we have one hundred twenty six divided by 9 equals 14 because nine multiplied by fourteen equals one hundred twenty six. We had nine fourteen times as a result of our sharing."
Ms. Davis wrote some problems for the children to practice their thinking.
1. Solve the following. What key facts will help you?
a. 104 / 8 = ? ↔ 8 x ? = 104
b. 112 / 7 = ? ↔ 7 x ? = 112
c. 162 / 9 = ? ↔ ? x 9 = 162
d. 102 / 6 = ? ↔ ? x 6 = 102
Make up a situation for each number sentence above.
2. Solve
a. 108 / 9 = ? b. 105 / 7 = ? c. 112 / 8 = ? d. 144 / 8 = ?
e. 114 / 6 = ? f. 153 / 9 = ? g. 126 / 6 = ? h. 144 / 18 = ?
Ms. Davis is often asked, "Will they ever use the traditional algorithm?"
Ms. Davis: The traditional algorithm tends to be a way of recording some steps that are not connected to student's conceptual understanding of division. Often it is misleading with the 'nine won't go into 1' process when it was really 100, and 9 certainly would go into 100! The recording done in my classroom is about representing student thinking about division.
Before the lesson ends, Ms. Davis suggests another way for them to record their thinking.
Ms. Davis wrote the following on the board:
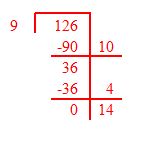
and spoke to the class.
"How does this recording relate to the way you solved the problem?"
Students were asked to discuss this in their groups and be ready to report back to the class. The sharing begins.....
"It is the same really."
"You shared out in sets of 10 as well, just like we did."
"Or it could have been 10 bags at a time, don't forget that. Both ways reduce the pile by 90."
"I know that 9 x 4 = 36. That makes 4 more bags."
"Ten bags and 4 bags make 14." We all got the same answer.
Resources
- Although "long division" is the primary (and often the only) strategy used in text books to teach division with larger numbers, it is heavily procedural and does not make sense to many students who use it including those who have "mastered" it. It is the teacher's responsibility to build understanding into the mathematics being performed.
- Students need the opportunity to explore division with larger numbers. Although you would not want them using manipulatives as their only way of solving problems, they are important to help establish the meaning of an operation.
For example, for the problem "8 students from a soccer team have a car wash to raise money for their uniforms and equipment. They earn $250. If they are splitting the earnings evenly, how much will each student get?"
Once students establish that the problem to be solved is 250 ÷ 8, choose 8 students to be the soccer players and give them $250 in fake dollar bills (giving the money in all ten dollar bills might be a good way to start. They could model dividing the money up with help from the audience while someone (another student or the teacher) makes notation of the mathematics on the board. When they have money left over, they trade it in for smaller bills or coins, until they each have the same amount, $31.25.
When modeling using different "real-life" materials and situations, division becomes more meaningful and accuracy becomes more important to students.
- It is important for students to share their problem solving strategy. Sharing provides students with the opportunity to talk through their thinking. As students listen to shared strategies they are able to learn from their peer, as well as ask questions, which furthers the thinking of both the student sharing and those listening.
- When students share strategies for solving problems, it is important for teachers to build connections between strategies. Students do not necessarily make these connections unless they are visible.
- Connecting multiplication and division is important since many students are more fluent with their multiplication facts and will be able to solve division by thinking multiplicatively.
- Encourage students to check division problem solutions using a related multiplication problem.
- Repeated subtraction is a valid strategy but includes many opportunities for errors.. Encourage more efficient strategies by pointing out the relationship between the "repeated subtraction" strategy and other strategies.
For example: To solve 250 ÷ 8 by repeatedly subtracting 8 would not only be time consuming, it would also be cumbersome and inefficient. But if a student could use 5 groups of 8, which would be 40, they could use the method with more accuracy and efficiency. It is also helpful to teach children how to keep track of their thinking. See the example below:
Problem being solved: # of groups of 8 subtracted
250 - 40 = 210 5 5 x 8 = 40
210 - 40 = 170 5
170 - 40 = 130 5
130 - 40 = 90 5
90 - 40 = 50 5
50 - 40 = 10 5
10 - 8 = 2 1
remainder of 2 31
Answer: 250 ÷ 8 = 31 r 2
- Good questions, and good listening, will help children make sense of the mathematics, build self-confidence and encourage mathematical thinking and communication. A good question opens up a problem and supports different ways of thinking about it. The best questions are those that cannot be answered with a "yes" or a "no."
Getting Started
What do you need to find out?
What do you know now? How can you get the information? Where can you begin?
What terms do you understand/not understan?
What similar problems have you solved that would help?
While Working
How can you organize the information?
Can you make a drawing (model) to explain your thinking? What are other possibilities?
What would happen if...?
Can you describe an approach (strategy) you can use to solve this?
What do you need to do next?
Do you see any patterns or relationships that will help you solve this?
How does this relate to...?
Why did you...?
What assumptions are you making?
Reflecting about the Solution
How do you know your solution (conclusion) is reasonable? How did you arrive at your answer?
How can you convince me your answer makes sense?
What did you try that did not work? Has the question been answered?
Can the explanation be made clearer?
Responding (helps clarify and extend their thinking)
Tell me more.
Can you explain it in a different way?
Is there another possibility or strategy that would work?
Is there a more efficient strategy?
Help me understand this part ...
(Adapted from They're Counting on Us, California Mathematics Council, 1995)
NCTM Illuminations
Lesson Name: Every Breath You Take
Lesson Objective: These activities help children to develop number sense through activities involving collection, representation, and analysis of data. In addition, children practice reading and writing large numbers and use estimation to arrive at appropriate answers.
Additional Instructional Resources
Duncan, N., Geer, C., Huinker, D., Leutzinger, L., Rathmell, E., & Thompson, C.(2007). Navigating through number and operations in grades 3-5. Reston, VA: National Council of Teachers of Mathematics.
Parrish, S. (2010). Number talks: Helping children build mental math and computation strategies grades K-5. Sausalito. CA: Math Solutions.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Van de Walle, J., Karp, K., Bay-Williams, J. (2010). Elementary and middle school mathematics: Teaching developmentally. (7th ed.) Boston, MA: Allyn & Bacon.
Van de Walle, J. & Lovin, L. (2006). Teaching student-centered mathematics grades 3-5. Boston, MA: Pearson Education.
"Vocabulary literally is the
key tool for thinking."
Ruby Payne
Mathematics vocabulary words describe mathematical relationships and concepts and cannot be understood by simply practicing definitions. Students need to have experiences communicating ideas using these words to explain, support, and justify their thinking.
Learning vocabulary in the mathematics classroom is contingent upon the following:
Integration: Connecting new vocabulary to prior knowledge and previously learned vocabulary. The brain seeks connections and ways to make meaning which occurs when accessing prior knowledge.
Repetition: Using the word or concept many times during the learning process and connecting the word or concept with its meaning. The role of the teacher is to provide experiences that will guarantee connections are made between mathematical concepts, relationships, and corresponding vocabulary words.
Meaningful Multiple and varied opportunities to use the words in context. These
Use: opportunities occur when students explain their thinking, ask clarifying questions, write about mathematics, and think aloud when solving problems. Teachers should constantly probing student thinking in order to determine if students are connecting mathematics concepts and relationships with appropriate mathematics vocabulary.
Strategies for vocabulary development
Students do not learn vocabulary words by memorizing and practicing definitions. The following strategies keep vocabulary visible and accessible during instruction.
Mathematics Word Bank: Each unit of study should have word banks visible during instruction. Words and corresponding definitions are added to the word bank as the need arises. Students refer to word banks when communicating mathematical ideas which leads to greater understanding and application of words in context.
Labeled pictures and charts: Diagrams that are labeled provide opportunities for students to anchor their thinking as they develop conceptual understanding and increase opportunities for student learning.
Frayer Model: The Frayer Model connects words, definitions, examples and non-examples.

Example/Non-example Charts: This graphic organizer allows students to reason about mathematical relationships as they develop conceptual understanding of mathematics vocabulary words. Teachers should use these during the instructional process to engage student in thinking about the meaning of words.
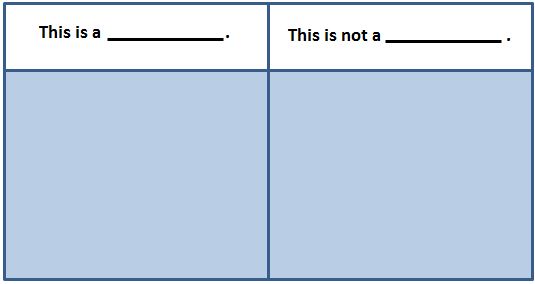
Vocabulary Strips: Vocabulary strips give students a way to organize critical information about mathematics vocabulary words.
|
word |
definition |
illustration |
Encouraging students to verbalize thinking by drawing, talking, and writing increases opportunities to use the mathematics vocabulary words in context.
Additional Resources for Vocabulary Development
Murray, M. (2004). Teaching mathematics vocabulary in context. Portsmouth, NH: Heinemann.
Sammons, L., (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
Reflection - Critical questions regarding the teaching and learning of these benchmarks:
What are the key ideas related to dividing multi-digit whole numbers by one- or two-digit numbers at the fourth grade level? How do student misconceptions interfere with mastery of these ideas?
What strategies might a fourth grader use when dividing multi-digit numbers by one- or two-digit numbers?
Examine student work related to a task involving division of a multi-digit number by a one- or two-digit number. What evidence do you need to say a student is proficient? Using three pieces of student work, determine what student understanding is observed through the work.
When checking for student understanding, what should teachers:
- listen for in student conversations?
- look for in student work?
- ask during classroom discussions?
How can teachers assess student learning related to these benchmarks?
How are these benchmarks related to other benchmarks at the fourth grade level?
Professional Learning Community Resources
Bamberger, H., Oberdorf, C., & Schultz-Ferrell, K.. (2010). Math misconceptions prek-grade 5: From misunderstanding to deep understanding. Portsmouth, NH: Heinemann.
Chapin, S. and Johnson, A. (2006). Math matters: Understanding the math you teach: Grades k-8, 2nd edition. Sausalito, CA: Math Solutions.
Chapin, S., O'Connor, C., & Canavan Anderson, N. (2009). Classroom discussions: Using math talk to help students learn (Grades K-6). Sausalito, CA: Math Solutions.
Dacey, L., & Lynch, J. (2007). Math for all: Differentiating instruction grades 3-5. Sausalito, CA: Math Solutions.
Fosnot, C., & Dolk, M.. (2002). Young mathematicians at work: Constructing multiplication and division. Portsmouth, NH: Heinemann.
Hyde, Arthur. (2006) Comprehending math adapting reading strategies to teach mathematics, K-6. Portsmouth, NH: Heinemann.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for elementary school teachers. Reston, VA: National Council of Teachers of Mathematics.
Otto, A., Caldwell, J., Wallus Hancock, S., & Zbiek, R..(2011). Developing essential understanding of multiplication and division for teaching mathematics in grades 3 - 5. Reston, VA.: National Council of Teachers of Mathematics.
Parrish, S. (2010). Number talks: Helping children build mental math and computation strategies grades K-5. Sausalito. CA: Math Solutions.
Sammons, L., (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
Schielack, J. (2009). Focus in Grade 4: Teaching with curriculum focal points. Reston, VA: National Council of Teachers of Mathematics.
Bamberger, H., Oberdorf, C., & Schultz-Ferrell, K. (2010). Math misconceptions prek-grade 5: From misunderstanding to deep understanding. Portsmouth, NH: Heinemann.
Bender, W. (2009). Differentiating math instruction: Strategies that work for k-8 classrooms! Thousand Oaks, CA. Corwin Press.
Bresser, R., Melanese, K., & Sphar, C. (2008). Supporting English language learners in math class, grades 3-5. Sausalito, CA: Math Solutions Publications.
Burns, M. (Ed) (1998). Leading the way: Principals and superintendents look at math instruction. Sausalito, CA: Math Solutions.
Cavanagh, M. (2004). Math to know: A mathematics handbook. Wilmington, MA: Great Source Education Group, Inc.
Chapin, S. and Johnson, A. (2006). Math Matters: Understanding the math you teach, grades K-8, 2nd Edition. Sausalito, CA: Math Solutions Press.
Donovan, S., & Bradford, J. (Eds). (2005). How students learn: Mathematics in the Classroom. Washington, DC: National Academies Press.
Felux, C., & Snowdy, P. (Eds.). (2006). The math coach field guide: Charting your course. Sausalito, CA: Math Solutions.
Fosnot, C., & Dolk, M. (2002). Young mathematicians at work: Multiplication and division. Portsmouth, NH: Heinemann.
Kilpatrick, J., & Swafford, J. (Eds). (2001). Adding it up: Helping children learn mathematics. Washington, DC: National Academies Press.
Leinwand, S., (2000). Sensible mathematics: A guide for school leaders. Portsmouth, NH: Heinemann.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for elementary school teachers. Reston, VA: National Council of Teachers of Mathematics.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for school administrators. Reston, VA: National Council of Teachers of Mathematics.
Murray, M. (2004). Teaching mathematics vocabulary in context. Portsmouth, NH: Heinemann.
Murray, M. & Jorgensen, J. (2007). The differentiated math classroom: A guide for teachers k-8. Portsmouth, NH: Heinemann.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA.: National Council of Teachers of Mathematics.
O'Connell, S., & SanGiovanni, J. (2011). Mastering the basic math facts: Multiplication and division, strategies, activities & interventions to move students beyond memorization. Portsmouth, NH: Heinemann.
Otto, A., Caldwell, J., Wallus Hancock, S., & Zbiek, R. (2011). Developing essential understanding of multiplication and division for teaching mathematics in grades 3 - 5. Reston, VA.: National Council of Teachers of Mathematics.
Parrish, S. (2010). Number talks: Helping children build mental math and computation strategies grades K-5. Sausalito. CA: Math Solutions.
Sammons, L., (2011). Building Mathematical Comprehension-Using Literacy Strategies to Make Meaning. Huntington Beach, CA: Shell Education.
Schielack, J., Charles, R., Clements, D., Duckett, P., Fennell, F., Lewandowski, S., Trevino, E., & Zbiek, R. M. (2006). Curriculum focal points for prekindergarten through grade 8 mathematics: A quest for coherence. Reston, VA.: National Council of Teachers of Mathematics.
Schielack, J. (2009). Focus in grade 4: Teaching with curriculum focal points. Reston, VA: National Council of Teachers of Mathematics.
Seeley, C. (2009). Faster isn't smarter: Messages about math teaching and learning in the 21st century. Sausalito, CA: Math Solutions.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Van de Walle, J., Karp, K., Bay-Williams, J. (2010). Elementary and middle school mathematics: Teaching developmentally. (7th Ed.) Boston, MA: Allyn & Bacon.
Van de Walle, J. & Lovin, L. (2006). Teaching student-centered mathematics grades 3-5. Boston, MA: Pearson Education.
West, L., & Staub, F. (2003). Content focused coaching: Transforming mathematics lessons. Portsmouth, NH: Heinemann.
Assessment
1. 908 ÷ 4 =
A. 202 B. 212 C. 227 D. 247
Solution: C: 227
Benchmark: 4.1.1.6 Source: MCAIII Sample Items
2. There are 35 students going on a class trip. The students ride in vans. There are 7 students riding in each van. How many vans are needed to take all the students?
A. 4 B. 5 C. 6 D. 7
Solution: B: 5
Benchmark: 4.1.1.6 Source: MCAIII Sample Items MCAIII: Answer: B. 5
3. Kira is using 1-inch square tiles to cover a table top. The table top is 24 inches long and 18 inches wide. She lays the tiles into strips of 6. How many strips of tile will Kira need to cover the table with no gaps?
A. 14 B. 18 C. 72 D. 432
Solution: C: 72
Benchmark: 4.1.1.6 Source: MCAIII Sample Items
4. A camping group bought 15 sleeping bags that cost $42 each and a tent that cost $160. What was the total cost of the sleeping bags and the tent?
A. $217 B. $630 C. $790 D. $2,442
Solution: C: $790
Benchmark: 4.1.1.6 Source: MCAIII Sample Items
4. All of the fourth grade classrooms at Pine Elementary are going on a field trip. There are 28 students in each of the 6 classes. If the students sit three to a seat, how many seats will they fill?
Solution: 56 (28 x 6) ÷ 3 = 56
Benchmark: 4.1.1.6
5. Terry divided 65 by 7 and came up with the answer 9.3; verify or prove the answer is wrong using a related multiplication problem.
- Possible solution: 7x9=63; 7x.3=2.1 and 63+2.1=65.1. The problem was 65 divided by 7 not 65.1 divided by 7. Terry is wrong.
Benchmark: 4.1.1.6
- Possible Solution: Terry is wrong because 7x9=63, and 65-63=2, the answer to 65/7 is 9 remainder 2.
Benchmark: 4.1.1.6
Differentiation
Students who struggle with multi-digit division need to revisit the division of a two-digit number by a one-digit number. This must involve the use of concrete models including arrays and sets of objects. When models are used students need to see them connected to a recording. In addition, these students need to think about types of division problems - problems that involve finding the number in equal groups and problems that involve finding the number of groups. Recognition of these problem types will influence solution strategies. The connection between division and subtraction needs to be emphasized.
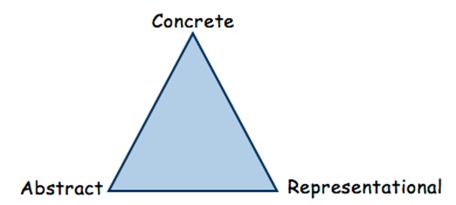
Concrete - Representational - Abstract Instructional Approach
The Concrete-Representational-Abstract Instructional Approach (CRA) is a research-based instructional strategy that has proven effective in enhancing the mathematics performance of students who struggle with mathematics.
The CRA approach is based on three stages during the learning process:
Concrete - Representational - Abstract
The Concrete Stage is the doing stage. The concrete stage is the most critical in terms of developing conceptual understanding of mathematical skills and concepts. At this stage, teachers use manipulatives to model mathematical concepts. The physical act of touching and moving manipulatives enables students to experience the mathematical concept at a concrete level. Research shows that students who use concrete materials develop more precise and comprehensive mental representations, understand and apply mathematical concepts, and are more motivated and on-task. Manipulatives must be selected based upon connections to the mathematical concept and the students' developmental level.
The Representational Stage is the drawing stage. Mathematical concepts are represented using pictures or drawings of the manipulatives previously used at the Concrete Stage. Students move to this level after they have successfully used concrete materials to demonstrate conceptual understanding and solve problems. They are moving from a concrete level of understanding toward an abstract level of understanding when drawing or using pictures to represent their thinking. Students continue exploring the mathematical concept at this level while teachers are asking questions to elicit student thinking and understanding.
The Abstract Stage is the symbolic stage. Teachers model mathematical concepts using numbers and mathematical symbols. Operation symbols are used to represent addition, subtraction, multiplication and division. Some students may not make a clean transfer to this level. They will work with some symbols and some pictures as they build abstract understanding. Moving to the abstract level too quickly causes many student errors. Practice at the abstract level will not lead to increased understanding unless students have a foundation based upon concrete and pictorial representations.
Additional Resources
Bender, W. (2009). Differentiating math instruction: Strategies that work for k-8 classrooms! Thousand Oaks, CA.: Corwin Press.
Dacey, L., & Lynch, J. (2007). Math for all: Differentiating instruction grades 3-5. Sausalito, CA: Math Solutions.
Murray, M. & Jorgensen, J. (2007). The differentiated math classroom: A guide for teachers k-8. Portsmouth, NH: Heinemann.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Van de Walle, J., Karp, K., Bay-Williams, J. (2010). Elementary and middle school mathematics: Teaching developmentally. (7th ed.) Boston, MA: Allyn & Bacon.
Van de Walle, J. & Lovin, L. (2006). Teaching student-centered mathematics grades 3-5. Boston, MA: Pearson Education.
Work in small groups to solve problems.
In working with the concept of division, small group work allows more opportunity to share and model thinking, to ask questions, and to justify thinking. It also gives students the chance to hear other explanations and make connections between solution strategies. The use of appropriate vocabulary during group work connects vocabulary to concept development.
Use vocabulary specific to the task.
This entails saying the words in relationship to the numbers, the operations and the steps involved. It also includes labeling the mathematics on the board with the correct terminology. This helps with specific vocabulary acquisition. When avoiding the use of correct terminology students do not hear it in context and have little chance of learning and applying the vocabulary. Using the word "dividend" and saying, "This is the number we are dividing into smaller groups" and then labeling it as such gives students a chance to attach meaning to the word "dividend." It also helps to pre-teach the vocabulary to students and give them a labeled example to have with them as the whole group is learning, and to refer to as they work in their small groups.
- Word banks need to be part of the student learning environment in every mathematics unit of study.
- Use vocabulary graphic organizers such as the Frayer model (see below) to emphasize vocabulary words count, first, second, third, etc.
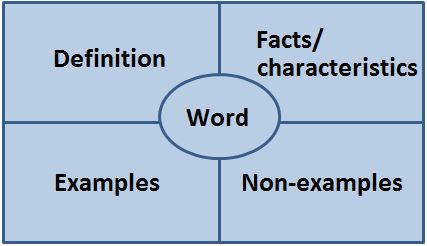
- Sentence Frames
Math sentence frames provide support that English Language Learners need in order to fully participate in math discussions. Sentence frames provide appropriate sentence structure models, increase the likelihood of responses using content vocabulary, help students to conceptualize words and build confidence in English Language Learners.
Sample sentence frames related to these benchmarks:
|
In this division problem, I need to find _____________________________________. |
|
This is how I solved the problem. First, ____________________________________. Second, __________________________________. . . . Last ______________________________________. |
|
I know the number of groups, I have to find the _____________________________. |
|
I know the number in each group. I have to find the __________________________. |
- When assessing the math skills of an ELL student it is important to determine if the student has difficulty with the math concept or with the language used to describe the concept and conceptual understanding.
Additional ELL Resources
Bresser, R., Melanese, K., & Sphar, C. (2008). Supporting English language learners in math class, grades k-2. Sausalito, CA: Math Solutions Publications.
Compare and contrast student solution strategies for division with solution strategies used in other parts of the world.
Additional Resources
Bender, W. (2009). Differentiating math instruction: Strategies that work for k-8 classrooms! Thousand Oaks, CA.: Corwin Press.
Dacey, L., & Lynch, J. (2007). Math for all: Differentiating instruction grades 3-5. Sausalito, CA: Math Solutions.
Murray, M. & Jorgensen, J. (2007). The differentiated math classroom: A guide for teachers k-8. Portsmouth, NH: Heinemann.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Parents/Admin
Administrative/Peer Classroom Observation
|
Students are: (descriptive list) |
Teachers are: (descriptive list) |
|
using models when solving multi-step problems involving division. |
checking for student understanding of the multi-step problems. |
|
solving division problems and interpreting the answers based on the type of problem being solved, for example, does it make sense to have the remainder translated into a fraction? No if it is people, but yes if it is inches. |
providing both "how many groups" and "how many in each group" division problems. Discussing both types with students and expecting students to identify these problem types. |
|
sharing strategies for solving division problems using both numeric notation and verbal or written explanations of the steps taken. |
evaluating strategies for accuracy, efficiency and ability to be replicated.
asking questions about steps that are not clear, seem redundant or have been missed. |
|
making connections between different strategies. Looking for similarities such as partial products, expanded notation, and repeated subtraction. |
helping to identify similar steps in different strategies using specific vocabulary and correct place value notation in the "traditional algorithm" 3 r For example: 8 │250 -240 not just "24 and 10 bring down the zero" |
|
checking their working in division by multiplying the divisor and the quotient (and adding the remainder if needed) to see if they arrive at the original dividend. |
teaching why and how to check their answers, reinforcing the idea that division and multiplication are reciprocal operations |
What should I look for in the mathematics classroom?
(Adapted from SciMathMN,1997)
What are students doing?
- Working in groups to make conjectures and solve problems.
- Solving real-world problems, not just practicing a collection of isolated skills.
- Representing mathematical ideas using concrete materials, pictures and symbols. Students know how and when to use tools such as blocks, scales, calculators, and computers.
- Communicating mathematical ideas to one another through examples, demonstrations, models, drawing, and logical arguments.
- Recognizing and connecting mathematical ideas.
- Justifying their thinking and explaining different ways to solve a problem.
What are teachers doing?
- Making student thinking the cornerstone of the learning process. This involves helping students organize, record, represent, and communicate their thinking.
- Challenging students to think deeply about problems and encouraging a variety of approaches to a solution.
- Connecting new mathematical concepts to previously learned ideas.
- Providing a safe classroom environment where ideas are freely shared, discussed and analyzed.
- Selecting appropriate activities and materials to support the learning of every student.
- Working with other teachers to make connections between disciplines to show how math is related to other subjects.
- Using assessments to uncover student thinking in order to guide instruction and assess understanding.
Additional Resources
For Mathematics Coaches
Chapin, S. and Johnson, A. (2006). Math matters: Understanding the math you teach: Grades k-8, 2nd edition. Sausalito, CA: Math Solutions.
Donovan, S., & Bradford, J. (Eds). (2005). How students learn: Mathematics in the classroom. Washington, DC: National Academies Press.
Felux, C., & Snowdy, P. (Eds.). (2006). The math coach field guide: Charting your course. Sausalito, CA: Math Solutions.
Sammons, L., (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
West, L., & Staub, F. (2003). Content focused coaching: Transforming mathematics lessons. Portsmouth, NH: Heinemann.
For Administrators
Burns, M. (Ed). (1998). Leading the way: Principals and superintendents look at math instruction. Sausalito, CA: Math Solutions.
Kilpatrick, J., & Swafford, J. (Eds). (2001). Adding it up: Helping children learn mathematics. Washington, DC: National Academies Press.
Leinwand, S. (2000). Sensible mathematics: A guide for school leaders. Portsmouth, NH: Heinemann.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for school administrators. Reston, VA: National Council of Teachers of Mathematics.
Seeley, C. (2009). Faster isn't smarter: Messages about math teaching and learning in the 21st century. Sausalito, CA: Math Solutions.
Mathematics handbooks to be used as home references:
Cavanagh, M. (2004). Math to Know: A mathematics handbook. Wilmington, MA: Great Source Education Group, Inc.
Cavanagh, M. (2006). Math to learn: A mathematics handbook. Wilmington, MA: Great Source Education Group, Inc.
Helping your child learn mathematics
Provides activities for children in preschool through grade 5
What should I look for in the mathematics program in my child's school? A Guide for Parents developed by SciMathMN
Help Your Children Make Sense of Math
Ask the right questions
In helping children learn, one goal is to assist children in becoming critical and independent thinkers. You can help by asking questions that guide, without telling them what to do.
Good questions, and good listening, will help children make sense of the mathematics, build self-confidence and encourage mathematical thinking and communication. A good question opens up a problem and supports different ways of thinking about it. The best questions are those that cannot be answered with a "yes" or a "no."
Getting Started
What do you need to find out?
What do you know now? How can you get the information? Where can you begin?
What terms do you understand/not understand?
What similar problems have you solved that would help?
While Working
How can you organize the information?
Can you make a drawing (model) to explain your thinking? What are other possibilities?
What would happen if . . . ?
Can you describe an approach (strategy) you can use to solve this?
What do you need to do next?
Do you see any patterns or relationships that will help you solve this?
How does this relate to ...?
Can you make a prediction?
Why did you...?
What assumptions are you making?
Reflecting about the Solution
How do you know your solution (conclusion) is reasonable? How did you arrive at your answer?
How can you convince me your answer makes sense?
What did you try that did not work?
Has the question been answered?
Can the explanation be made clearer?
Responding (helps clarify and extend their thinking)
Tell me more.
Can you explain it in a different way?
Is there another possibility or strategy that would work?
Is there a more efficient strategy?
Help me understand this part...
Adapted from They're counting on us, California Mathematics Council, 1995
