8.1.1A Rational, Irrational & Real Numbers
Classify real numbers as rational or irrational. Know that when a square root of a positive integer is not an integer, then it is irrational. Know that the sum of a rational number and an irrational number is irrational, and the product of a non-zero rational number and an irrational number is irrational.
For example: Classify the following numbers as whole numbers, integers, rational numbers, irrational numbers, recognizing that some numbers belong in more than one category: $\frac{6}{3},\frac{3}{6},3.\bar{6},\frac{\pi }{2},-\sqrt{4},\sqrt{10},-6.7$.
Compare real numbers; locate real numbers on a number line. Identify the square root of a positive integer as an integer, or if it is not an integer, locate it as a real number between two consecutive positive integers.
For example: Put the following numbers in order from smallest to largest: $2,\sqrt{3},-4,-6.8,-\sqrt{37}$.
Another example: $\sqrt{68}$ is an irrational number between 8 and 9.
Determine rational approximations for solutions to problems involving real numbers.
For example: A calculator can be used to determine that $\sqrt{7}$ is approximately 2.65.
Another example: To check that $1\frac{5}{12}$ is slightly bigger than $\sqrt{2}$, do the calculation $\left ( 1\frac{5}{12} \right )^{2}=\left ( \frac{17}{12} \right )^{2}=\frac{289}{144}=2\frac{1}{144}$.
Another example: Knowing that $\sqrt{10}$ is between 3 and 4, try squaring numbers like 3.5, 3.3, 3.1 to determine that 3.1 is a reasonable rational approximation of $\sqrt{10}$.
Overview
Standard 8.1.1 Essential Understandings
The focus of instruction in this standard is on understanding the real number system. Students have only experienced rational numbers in the form of whole numbers, integers, decimals and fractions. Students will expand their understanding of rational numbers as they represent and operate with very large or very small numbers in scientific notation and exponential form. Students complete their understanding of the real number system as they are introduced to irrational numbers. To best understand this new category of real numbers, students need to make comparisons between rational and irrational numbers. By making comparisons, students will develop the understanding of the unique characteristics of each. Typically, the only irrational number students are familiar with is the number pi. However, students have not necessarily connected pi with the characteristics of irrational numbers. Students need to identify the characteristics of irrational numbers as they compare to the rational number system. As students gain more familiarity with irrational numbers, they will be able to estimate the value and create meaning of irrational number solutions as they solve problems involving all real numbers.
Benchmark Group A - Rational, Irrational, and Real Numbers
8.1.1.1 Classify real numbers as rational or irrational. Know that when a square root of a positive integer is not an integer, then it is irrational. Know that the sum of a rational number and an irrational number is irrational, and the product of a non-zero rational number and an irrational number is irrational.
For example: Classify the following numbers as whole numbers, integers, rational numbers, irrational numbers, recognizing that some numbers belong in more than one category: $\frac{6}{3}, \frac{3}{6}, 3.\overline{6}, \frac{\pi }{2}, -\sqrt{4}, \sqrt{10}, -6.7$
Allowable notation: $\sqrt{18}$
8.1.1.2 Compare real numbers; locate real numbers on a number line. Identify the square root of a positive integer as an integer, or if it is not an integer, locate it as a real number between two consecutive positive integers.
For example: Put the following numbers in order from smallest to largest:
$2, \sqrt{3}, -4, -6.8, -\sqrt{37}$
Another example: $\sqrt{68}$ is an irrational number between 8 and 9.
Allowable notation: $\sqrt{18}$
8.1.1.3 Determine rational approximations for solutions to problems involving real numbers.
For example: A calculator can be used to determine that $\sqrt{7}$ is approximately 2.65.
Another example: To check that $1\frac{5}{8}$ is slightly bigger than $\sqrt{2}$, do the calculation $\left ( 1\frac{5}{12} \right )^{2}=\left ( \frac{17}{12} \right )^{2}=\frac{289}{144}=2\frac{1}{144}$.
Another example: Knowing that $\sqrt{10}$ is between 3 and 4, try squaring numbers like 3.5, 3.3, 3.1 to determine that 3.1 is a reasonable rational approximation of $\sqrt{10}$.
What students should know and be able to do [at a mastery level] related to these benchmarks:
- Compare real numbers;
- Classify numbers as rational or irrational;
- Locate rational and irrational number on a number line;
- Estimate the value of irrational numbers;
- Make rational approximations of square roots without using their calculator;
- Understand that adding or multiplying a rational number by an irrational number will create an irrational number.
Work from previous grades that supports this new learning includes:
- Identify the characteristics of rational numbers;
- Operate with rational numbers;
- Understand the meaning of a repeating decimal;
- Convert between decimals and fractions;
- Estimate the value of a fraction without the use of a calculator;
- Work with the Pythagorean Theorem and find the lengths that are square root values. (This is learned in 8th grade but is an important connection to this benchmark.)
NCTM Standards
Number and Operations
- Develop an understanding of large numbers and recognize and appropriately use exponential, scientific, and calculator notation.
- Understand and use the inverse relationships of addition and subtraction, multiplication and division, and squaring and finding square roots to simplify computations and solve problems.
- Compare and order fractions, decimals, and percents efficiently and find their approximate locations on a number line;
Common Core State Standards (CCSS)
Number Systems: Know that there are numbers that are not rational, and approximate them by rational numbers.
- 8.NS.1. Understand informally that every number has a decimal expansion; rational numbers have decimal expansions that terminate in 0s or eventually repeat, and conversely.
- 8.NS.2. Use rational approximations of irrational numbers to compare the size of irrational numbers, locate them approximately on a number line diagram, and estimate the value of expressions (e.g., (pi)^2). For example, by truncating the decimal expansion of sqrt2 (square root of 2), show that sqrt2 is between 1 and 2, then between 1.4 and 1.5, and explain how to continue on to get better approximations.
Expressions and Equations: Work with Radicals and Integer Exponents.
- 8.EE.1. Know and apply the properties of integer exponents to generate equivalent numerical expressions. For example, 3^2 × 3^(-5) = 3^(-3) = 1/(3^3) = 1/27.
- 8.EE.2. Use square root and cube root symbols to represent solutions to equations of the form x^2 = p and x^3 = p, where p is a positive rational number. Evaluate square roots of small perfect squares and cube roots of small perfect cubes. Know that sqrt2 is irrational.
- 8.EE.3. Use numbers expressed in the form of a single digit times an integer power of 10 to estimate very large or very small quantities, and to express how many times as much one is than the other. For example, estimate the population of the United States as 3 × 10^8 and the population of the world as 7 × 10^9, and determine that the world population is more than 20 times larger.
- 8.EE.4. Perform operations with numbers expressed in scientific notation, including problems where both decimal and scientific notation are used. Use scientific notation and choose units of appropriate size for measurements of very large or very small quantities (e.g., use millimeters per year for seafloor spreading). Interpret scientific notation that has been generated by technology.
Misconceptions
- Students often lose the meaning of the value of square roots.
- Students think any number that "goes on forever" is an irrational number.
- Students divide by 2 instead of taking the square root of a number. Most commonly, students simplify the $\sqrt{2}$ to be 1.
- Students think that irrational numbers are not relevant but are just another math thing to memorize.
- Students assume a square root is always irrational.
- Students mistake $\frac{22}{7}$ as an irrational number because they believe that $\frac{22}{7}=\pi $ instead of realizing that $\frac{22}{7}$ is an approximation for $\pi $ .
- When looking at radicals of fractions, students often assume that the number is irrational because the fraction is not a perfect square of an integer.
Vignette
In the Classroom
This number line activity gives students the chance to work with rational and irrational numbers.
Teacher: We have been doing a lot of work finding the length of the hypotenuse on a variety of right triangles. Let's look at a few right triangles. Find the missing side length.
What do you notice about all the missing sides?
Student: They are all square roots.
Teacher: So what is the actual length of each missing side?
Student: Well, just put the square root of 8 in your calculator and you get 2.828427....
Teacher: Is that an exact value?
Student: Well, no, but it's close enough. Isn't it?
Teacher: When you estimate the value on your calculator, it's called a rational approximation. The rational approximation helps us make sense out of the irrational number. We need to talk about this type of numbers. If we have a square root number that is not "perfect" or isn't equivalent to an integer, it fits in the category of irrational numbers. Anyone know anything about irrational numbers?
Student: Isn't pi an irrational number?
Teacher: Yes, pi is probably the most famous irrational number you are familiar with. So what makes pi special?
Student: It goes on and on forever without a repeating decimal pattern.
Teacher: That is what makes irrational numbers special. They go on forever without a repeating pattern. Can you think of any other irrational numbers?
Student: Not really.... I guess any square root like you said, like the square root of 50 or the square root of 34 or the square root of any number that isn't a perfect square.
Teacher: Ok, but you could also make up your own. What about 0.01001000100001000001...?
Student: But that's a pattern.
Teacher: Is it a repeating pattern?
Student: No, I guess not....
Teacher: As long as the number cannot be written as a fraction we have an irrational number. Talk with the person next to you and come up with your own irrational number. What did you come up with?
Group 1: 0.12123123412345....
Group 2: 0 .989796959493929190
Group 3: 0.23242526272829202123242526....
Group 4: 0.121122111222111122221111122222....
Teacher: OK. Let's look at these. Are all of these irrational?
Student: Group 2 doesn't have dots after so doesn't that mean the number ends and that it would not go on forever? So it's not irrational. We could write it as a fraction. It would be a really hard fraction to read but we could do it.
Teacher: So those dots do represent a number that goes on forever. If Group 2 put dots after it, would it be irrational?
Student: I guess. There is no repeating pattern yet so I think it would be good.
Student: I think Group 3's number is not irrational either. It has the dots but it started repeating; after 21, it went back to 232425 and so on.
Teacher: What about Group 1 and 4?
Student: Well, they both have patterns, but it's not a repeating pattern so I guess they would be irrational.
Teacher: If irrational numbers go on forever without a repeating pattern, how would we describe rational numbers?
Student: Everything else?
Teacher: So what is everything else?
Student: Whole numbers.
Student: Negative numbers.
Student: Fractions.
Student: Decimals that end or have a repeating pattern.
Teacher: I think you covered it. Let's see practice and sort this list of numbers. Split them into two categories: Rational and Irrational.
$-2, 2.4, \sqrt{12}, 7.1, \frac{-5}{6}, .\overline{3}, .1011011101111..., \sqrt{32}, 5.\overline{12}, \sqrt{8}, \frac{22}{7}, \sqrt{36},$ $-2.234234234...,$ $1.2657,$ $\frac{1}{9},$ $\frac{2}{-5}, -2.2, \sqrt{25}, -\sqrt{5}, \frac{3}{10}$

Teacher: How do your lists compare? Did you all agree?
Teacher: Now I have another task for you. You all need to be able to order numbers. Let's start with the rational list. I will give each group a card with one of the rational numbers from the list. You need to send one person up to the number line and clothes pin your number in the correct place on the line. Notice the number line already has -3, -2, -1, 0, 1, 2, 3, 4, 5, 6 labeled. Any questions?
Teacher: When you get your number, without using a calculator, agree as a group as to where it goes on the line.
Students place numbers on number line. Students do this relatively quickly and correctly.
Student: That was easy. We know how to order numbers.
Teacher: How did you figure out where the fractions went? I heard many groups wanting to use their calculator to find the decimal equivalent.
Student: We figured out that we really didn't have to figure out the exact value. We could just see where they were compared to others. We could figure out which integers they were in between and then try and figure out which it was closer to.
Teacher: So you had a strategy that worked when you couldn't find the decimal value. I'm glad to see that you were using some estimations to figure out approximately where to place the number. Remember these strategies because they will help your group as you place the irrational numbers on the number line.
Next, you will place the list of irrational numbers on the number line. Again each group will get a card and you will need to find the location of each irrational number without using the square root key on your calculator. Any questions?
Student: I can place the irrational numbers that are already in decimal form, but our group got $\sqrt{12}$. We have no idea the value of the decimal approximation.
Teacher: Let's do this one together. What do you know?
Student: It needs to be a number that when multiplied by itself is 12.
Teacher: That's a start. What are some possibilities?
Student: Well 3 × 3 is 9. So it has to be bigger than 3.
Student: And 4 × 4 is 16. So it has to be smaller than 4.
Student: Cool. That's kind of how we were figuring out the fractions. But now what? Where are we going to place it between 3 and 4?
Teacher: Let's try and compare all the numbers in square root form.
Consider what you just mentioned. I can write 3 as $\sqrt{9}$. I can write 4 as $\sqrt{16}$.
Where does $\sqrt{12}$ fit?
Student: If you compare 9, 12 and 16, 12 is almost right in the middle but a little closer to 9. There is a difference of 3 between 12 and 9 and a difference of 4 between 12 and 16.
Teacher: What would your rational approximation be for the $\sqrt{12}$?
Student: I would say 3.4 because it's almost in the middle but a little closer to 3.
Teacher: You just said 12 was closer to 9. So where did you get 3.4?
Student: I really meant that $\sqrt{12}$ is a little closer to the $\sqrt{9}$. And the $\sqrt{9}$ is 3.
Teacher: How could you check to see if your approximation is close?
Student: I would take 3.4 × 3.4 which is 11.56. That's not very close. But if I move to 3.5 that's too much. Maybe 3.45? That's better: 11.9025. But still not exact.
Teacher: Will we ever get exact?
Student: No, because it's an irrational number. The decimal place will go on forever.
Student: What about 3.457 times itself? That's 11.950849. I'm getting closer... How do I know when to stop?
Teacher: It's kind of like what you were doing with your fractions earlier. Depending on how accurate you need to be, you could just find how it compares to another number. It might be OK to know that it's almost halfway between 3 and 4 but a little bit close to 3. You might need to go further if you are comparing it to a number that is close to that value.
Teacher: Let's try another one before I send you off to locate your irrational number on the number line. Let's try $\sqrt{8}$. What do we know?
Student: Something times itself has to equal 8.
Student: 2 × 2 is 4 so I have to go bigger.
Student: 3 × 3 is 9 so I have to go smaller.
Student: So $\sqrt{8}$ is between 2 and 3.
Teacher: This is an important connection. If you can identify the two integers your square root is between you are making progress. Keep using those perfect squares as benchmarks. Where do we go from here?
Student: We have to look at where $\sqrt{8}$ is compared to 2 and 3.
Teacher: How do we do that?
Student: Line up the $\sqrt{4}$ that we got from the 2x2 and the $\sqrt{9}$ that we got from the 3x3 and then see where $\sqrt{8}$ fits in.
Student: I think it's closer to $\sqrt{9}$ so I estimate 2.7.
Student: I agree it's closer to $\sqrt{9}$ , but I say it's really close like 2.9 because 8 and 9 are only 1 away from each other.
Teacher: Anyone want to take 2.8? Let's test them. 2.7 × 2.7 gives us 7.29. 2.9 × 2.9 gives us 8.41. Someone make a better estimate based on what we know about 2.7 and 2.9.
Student: They are both off by a lot. It looks like 2.7 is off by the most so it should be closer to 2.9. I am going with 2.85. When I multiply it by itself, I get 8.1225. Shoot, it's still too much. But I am much closer.
Teacher: Could we make a better guess?
Student: I think 2.8 will be too small because we are so close. I'd go with 2.83 and get 8.0089. That's really close. I would be happy with that.
Teacher: Your turn to do it on your own. Figure out your rational approximation and locate it on the number line.
Resources
Teacher Notes
- Help students make meaning out of square roots by comparing them to perfect square roots and their rational value. This will help students make some meaning out of square roots that are irrational numbers.
- Connect irrational numbers to student learning about the Pythagorean Theorem. Students have already been working with square root values that are irrational numbers. This is an appropriate time to discuss the characteristics of irrational numbers and strategies to estimate their value.
- Connecting irrational numbers to their work with the Pythagorean Theorem and finding distances will help students see an application and understand the relevance of irrational square roots.
- Connecting pi to the area and circumference of a circle in context will help student see the relevance of irrational numbers.
- Have students make a list of the perfect square numbers and be familiar with them to be able to recognize that the square root of these numbers is an integer and therefore rational.
- Guide students to examine square roots of fractions and decimals as well to determine if the number is rational or irrational. ex $\sqrt{\frac{4}{9}}=\frac{2}{3}$ or $\sqrt{0.36}=0.6$ therefore these are both rational numbers.
- Learning about rational and irrational numbers might also be a good place to review inequalities. For example, let x be a real number and let 3 < x < 4. Name five rational values and 5 irrational values that x might have. Or find the integer value of x such that $\sqrt{37}\leq x\leq \sqrt{63}$. Answer: 7
Rational and irrational number line
This website offers directions for an activity that involves putting rational and irrational numbers in order on a number line.
Negatives on an everyday number line
This article that introduces a number line activity using benchmarks in students' own lives. It pushes them to think about the meaning of negative values and fractions and their placement on the number line. (Must be an NCTM member to view)
irrational number: a number which cannot be written as a fraction where the numerator and denominator are both integers and where the denominator does not equal zero; a non-repeating and non-terminating decimal number.
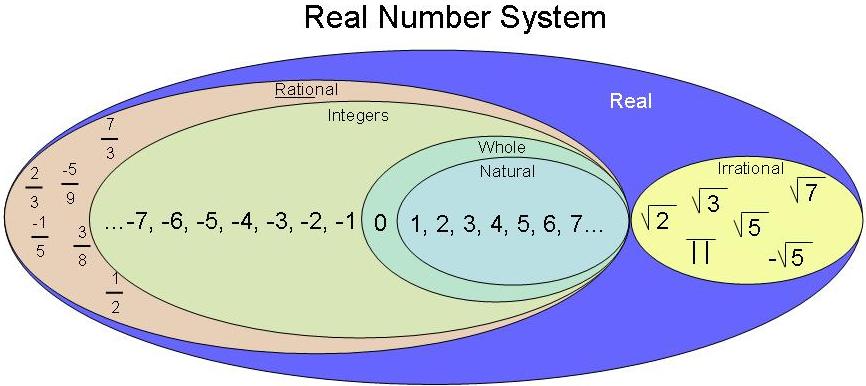
real number: a member of the set of naturals, wholes, integers, rational numbers, and irrational numbers.
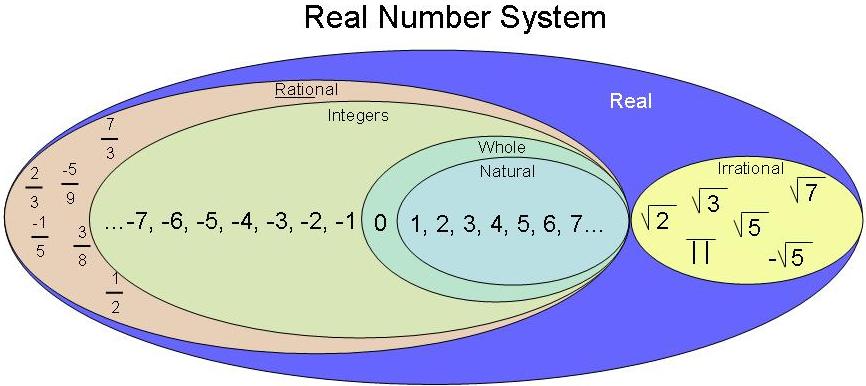
(Picture taken from Homework Help Secrets.)
square root: a divisor of a quantity that when squared gives the quantity.
Example: 5 is a square root of 25 because 5 × 5 = 25. Another square root of 25 is -5 because (-5) × (-5) = 25. The +5 is called the principle square root of 25.
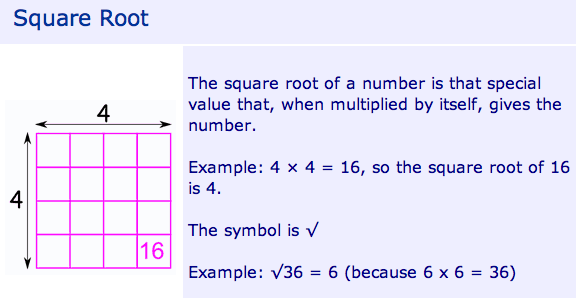
Radical: a root, such as $\sqrt{2}$, especially as indicated by a radical sign ($\sqrt{\ \ }$ ).
Reflection - Critical Questions regarding the teaching and learning of these benchmarks
- How have the students demonstrated that they understand the difference between rational and irrational numbers? How do you know?
- What student misconceptions have not been revealed/addressed?
- What teacher action(s) are needed to improve learning and performance?
- What student action(s) are needed to improve learning and performance?
- Exponents are not on the level. (n.d.). Mathstuff. Retrieved June 17, 2011, from http://www.mathnstuff.com/math/spoken/here/2class/210/laws.htm
- Heron's formula for the area of a triangle. (n.d.). Math Open Reference. Retrieved June 17, 2011, from http://www.mathopenref.com/heronsformula.html
- Illuminations: Resources for Teaching Math. (n.d.). Illuminations. Retrieved June 17, 2011, from http://illuminations.nctm.org/
- Irrational Numbers. (n.d.). Math is Fun. Retrieved June 17, 2011, from http://www.mathsisfun.com/irrational-numbers.html
- Multiplying and Dividing in Scientific Notation. (n.d.). Ohio Department of Education. Retrieved June 20, 2011, from http://ims.ode.state.oh.us/ODE/IMS/Lessons/Web_Content/CMA_LP_S01_BI_L0…
- Principles and standards for school mathematics. (2000). Reston, VA: National Council of Teachers of Mathematics.
- Pre-algebra review topic: Line-up cards. (n.d.). Oswego City School District Regents Exam Prep Center. Retrieved June 17, 2011, from http://regentsprep.org/Regents/math/ALGEBRA/AOP1/Tcards.htm
- Media Mill: Region 11 training: Multiplying by 10. (n.d.). CLA Media Mil : University of Minnesota. Retrieved June 19, 2011, from http://mediamill.cla.umn.edu/mediamill/display/75023
- Released Test Questions. (n.d.). California Department of Education. Retrieved June 17, 2011, from www.cde.ca.gov/ta/tg/sr/documents/cstrtqmath7.pdf
- Rules for Significant Figures. (n.d.). University of Wisconsin: Eau Claire. Retrieved June 19, 2011, from www.uwec.edu/carneymj/Adobe%20handouts/Significant%20Figures.pdf
- Schielack, J. F. (2010). Focus in grade 8: teaching with curriculum focal points. Reston, VA: National Council of Teachers of Mathematics.
- Scientific notation 2. (n.d.). Khan Academy. Retrieved June 19, 2011, from http://www.khanacademy.org/video/scientific-notation-2?playlist=Algebra…
- TAKS Released Tests. (n.d.). Texas Education Agency . Retrieved June 17, 2011, from http://www.tea.state.tx.us/student.assessment/taks/released-tests/
- Weidemann, W., Mikovch, A. K., & Hunt, J. B. (2001). Using a Lifeline to Give Rational Numbers a Personal Touch. Mathematics Teaching in the Middle School, 7(4), 210.
Assessment
1.
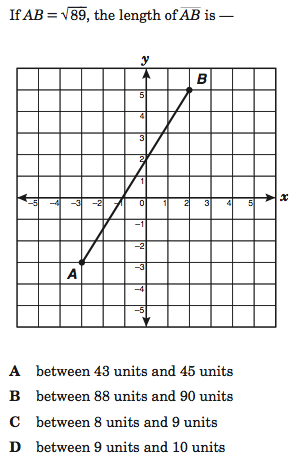
DOK Level: 1
Answer: d
Source: 2009 TAKS (Texas Assessment of Knowledge and Skills) grade 8 released test
2.

DOK Level: 1
Answer: d
Source: Grade 7 California standardized released test
3.

DOK Level: 1
Answer: d
Source: Minnesota MCA Item Sampler 8th grade
4.
Place the following real numbers on a number line: $\sqrt{4}, 0.4, -\frac{2}{3}, \sqrt{2},$ and $-1.5$
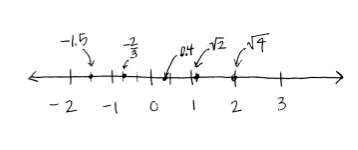
Answer: $-1.5, -\frac{2}{3}, 0.4, \sqrt{2}, \sqrt{4}$
DOK Level: 3
5. Which irrational number is the greatest?
- $\pi $
- Sqrt (10)
- Sqrt (5)
- Sqrt(8)
DOK Level: 1
Answer: b
Taken from this source
Differentiation
- Make a list of perfect squares to reference as students estimate the values of square roots.
- On the number line, label the integers above the line and the equivalent square root below the line.
- Have students write out the steps for estimating the value of the square root.
Example:
Find the two integers that $\sqrt{130}$ is between.
Find the perfect squares closest to 130.
81, 100, 121, 144
130 is between 121 and 144
121 < 130 < 144
$\sqrt{121}< \sqrt{130}< \sqrt{144}$
$11< \sqrt{130}< 12$
- Have the students prepare flash cards of the vocabulary terms for the different classifications of real numbers. Have them provide examples and non-examples with the definitions.
- Make a list of perfect squares to reference as students estimate the values of square roots.
- On the number line, label the integers above the line and the equivalent square root below the line.
- Have students make their own real number system diagram to reference.
- Find the exact value of n: $\left | \pi -3.14 \right |+\left | \pi -\frac{22}{7} \right |=n$
- Estimate values of cube roots.
- Heron's formula
Have students explore Heron's formula to find the area of a triangle.
- Have the students examine other famous irrational numbers other than $\pi $, such as e or the golden ratio phi (
 ).
).
Parents/Admin
|
Students are: (descriptive list) |
Teachers are: (descriptive list) |
|
ordering and comparing all real numbers on a number line. |
helping students make meaning out of square roots by comparing them to perfect square roots and their rational value; this will help students make meaning out of square roots that are irrational numbers. |
|
developing and discussing strategies to find the estimated value of an irrational number without a calculator. |
connecting irrational numbers to student learning about the Pythagorean Theorem. Students have already been working with square root values that are irrational numbers. Teachers are discussing the characteristics of irrational numbers and helping students develop strategies to estimate the value of the irrational numbers. |
|
solving problems in context with a real world connection. |
connecting irrational numbers to the students' work with the Pythagorean Theorem and finding distances to help students see the relevance of irrational square roots. |
|
connecting their learning of the Pythagorean Theorem to their understanding of irrational numbers. |
connecting pi to the area and circumference of a circle in context to help students see the relevance of irrational numbers
|
|
performing operations with real numbers and determining if the answers are rational or irrational. |
|
Parent Resources
