6.3.2A Angles & Intersecting Lines
Solve problems using the relationships between the angles formed by intersecting lines.
For example: If two streets cross, forming four corners such that one of the corners forms an angle of 120˚, determine the measures of the remaining three angles.
Another example: Recognize that pairs of interior and exterior angles in polygons have measures that sum to 180˚.
Overview
Standard 6.3.2 Essential Understandings
The focus of instruction at this level is to explore cause and effect relationships of angles in geometric figures. Relationships of complementary, supplementary, right, straight, adjacent and vertical angles formed by intersecting lines are used to solve problems. The relationship of the sum of the interior angles of a triangle is used to find missing angles in a triangle. This relationship is applied when students decompose other polygons into triangles to develop and use formulas for sums of their interior angles. While geometry is the focus, there are other connections occurring. The process of students developing understanding of these relationships and formula is a direct result of purposeful application of Algebraic Habits of Mind. Engaging students in doing and undoing, abstracting from numbers, and building rules are essential components of this learning process.
Benchmark Group A - Angles and Intersecting Lines
6.3.2.1 Solve problems using the relationships between the angles formed by intersecting lines.
What students should know and be able to do [at a mastery level] related to these benchmarks:
- Identify supplementary angles and know that the sum of the measures of these angles is 180o;
- Recognize straight angles and know that these angles measure 180o;
- Identify complementary angles and know that the sum of the measures of these angles is 90o;
- Know that right angles measure 90º;
- Recognize vertical (opposite) angles in a pair of intersecting lines and know that these angles are congruent;
- Determine the measure of all angles in a pair of intersecting lines when given the measure of one angle;
- Use the relationships formed by angles of intersecting lines to solve problems.
Work from previous grades that supports this new learning includes:
- Identify parallel and perpendicular lines in various contexts, and use them to describe and create geometric shapes, such as right triangles, rectangles, parallelograms and trapezoids;
- Measure angles in geometric figures and real-world objects with a protractor or angle ruler;
- Compare angles by size;
- Classify angles as acute, right and obtuse.
NCTM Standards
- Understand measurable attributes of objects and the units, systems, and processes of measurement:
- Understand, select, and use units of appropriate type to measure angles.
- Analyze characteristics and properties of two- and three-dimensional geometric shapes and develop mathematical arguments about geometric relationships:
- Understand relationships among types of two-dimensional objects using their defining properties;
- Investigate, describe, and reason about the results of subdividing and combining shapes.
- Use visualization, spatial reasoning, and geometric modeling to solve problems:
- Use geometric models to represent and explain numerical and algebraic relationships.
Common Core State Standards (CCSS)
- 7G Solve real-life and mathematical problems involving angle measure, area, surface area, and volume.
- 7.G.5. Use facts about supplementary, complementary, vertical, and adjacent angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure.
Misconceptions
- Students have difficulty distinguishing between angle as movement, as in rotation; angle as a geometric shape, a delineation of space by two intersecting lines; and angle as a measure.
- Students are unfamiliar with the symbolic notation used to identify angles and their measures.
- Students may use the point of intersection to name all angles formed by a pair of intersecting lines. For example, all angles formed by a pair of lines that intersect at point A may be referred to as angle A.
- Students confuse the terms supplementary and complementary.
- Students believe that complementary and supplementary angles must be adjacent.
- Students believe that all adjacent angles are either complementary or supplementary.
- Students may not recognize congruent angles because of the length of the rays in a drawing.
- Students may incorrectly identify vertical angles.
- Students may misread a protractor.
Vignette
In the following vignette, students at Worthington Middle School use their local map to explore angle relationships of intersecting lines.
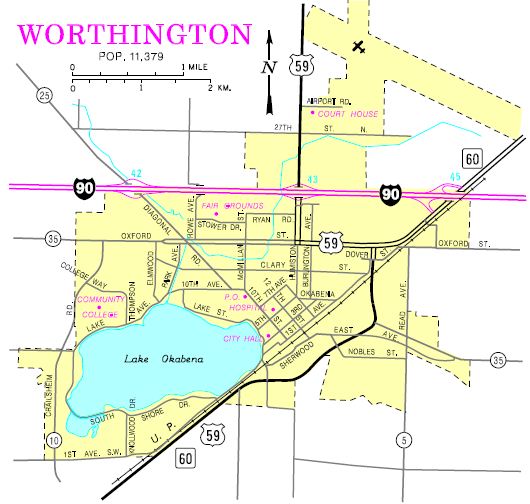
Teacher: Today we're going to explore the relationships of angles formed by intersecting lines. For this investigation, each of you will need a protractor, a map of our city, and your journal. Does everyone have their materials gathered? (Heads nod.) Let's get started then. Let's take a few minutes to get oriented to our maps. I don't see our school labeled on the map. Can anyone tell me where our school is located?
Student: We're on the southwest side of Lake Okabena. The address is 1401 Crailsheim Road. I think the school is somewhere close to where the sign for County Road 10 is drawn on Crailsheim Road.
Teacher: Let's all try to locate the place he's talking about. Can everyone find Crailsheim Road just to the left, or west of Lake Okabena? The sign for County Road 10 is just north of the intersection of Crailsheim Road and 1st Ave SW. Do you agree that that's where Worthington Middle School is located?
Student: Yeah, I think so. Our bus drives past the Community College on our way to school, so that makes sense.
Teacher: Our task today is to explore the relationship of the angles formed by intersecting lines. How can this map help us accomplish that?
Student: The roads are sort of like lines, and roads have intersections because that's where accidents usually happen.
Teacher: Good observation. It seems you have a clear understanding of what I mean by intersecting lines. They're lines that cross or meet. Where on the map do you see examples of intersecting lines?
Student: The intersections are everywhere. This map doesn't even show all of the intersections we have in Worthington. It only shows the intersections of main roads.
Teacher: Yes, only the main roads are shown on this map. In just a few minutes, you're going to use your protractor to measure the angles formed by intersecting roads, so perhaps it would be helpful to make a list of some of intersections that might be easier to measure on this map.
Student: Like Interstate 90 and Highway 25?
Teacher: Where do you see those roads?
Student: They're in the top left corner of the map. Highway 25 is the road that goes to the town of Reading.
Student: I see it. I think it would be easy to measure the angles where Interstate 90 and Highway 59 intersect.
Teacher: I agree. I'm going to begin a list with your suggestions of intersecting roads, or lines, to measure.
Student: How about the intersection of Interstate 90 and Diagonal Road? That's kinda funny. I've never thought about the name Diagonal Road before.
Teacher: What other suggestions for measuring do you have?
Student: Do we need to limit our measurement to just roads or can we measure the lines formed by the intersecting runways at the airport?
Teacher: We're looking for examples of intersecting lines. If the runways meet that criteria, let's measure the angles formed.
Student: I live at the corner of Diagonal Road and Lake Avenue. Can I measure those angles?
Teacher: Are Diagonal Road and Lake Avenue intersecting lines?
Student: Yes.
Teacher: Then go ahead and measure the angles formed. Let's get started with the task. I want each of you to choose three sets of intersecting lines and measure the angles formed by them. For some of the angles, you may find it helpful to extend the length of the sides, or rays, before measuring. Please make a drawing of each intersection in your journal and record your measurements. When you're finished, take a minute to look for patterns in the relationships of the angles, look for patterns and record your thoughts.
(Teacher circulates around the room assisting students.)
Sample student work:
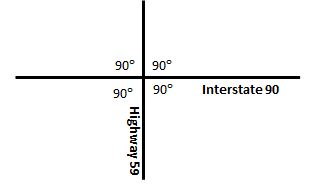
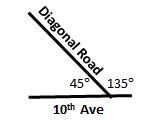
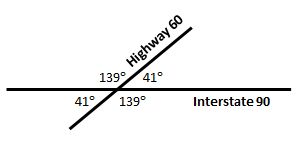
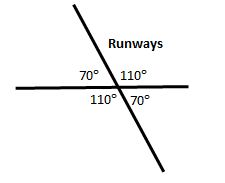
Teacher: What relationships did you notice in the angles formed by intersecting lines:
Student: I noticed that the angles that were across from each other always had the same measure.
Teacher: Can you give me an example?
Student: Like runways. One set of angles across from each other measured 110º and the other set of angles measured 70º and 70º.
Teacher: Did anyone else make that same observation?
Student: For Highway 60 and Interstate 90 I got 41º for one set of angles and 139º for the other set.
Student: Well, I didn't always get exactly the same measurement for both sets, but I was within two degrees.
Teacher: How do you explain that?
Student: I think that I was supposed to get the same measure for the angles across from each other, but my measuring was a little off.
Teacher: Yes, all measurements have some inaccuracy, but it sounds like you still saw the same pattern. What name do we give angles opposite, or across from each other in intersecting lines?
Student: I know! Vertical angles!
Teacher: Yes, angles formed by intersecting lines that are across from each other, or opposite, are called vertical angles. And what pattern did we discover about the measurement of vertical angles?
Student: They always have the same measure.
Teacher: Yes, vertical angles are always congruent, or have the same measure. Did anyone else have other observations?
Student: My lines didn't cross - they only met, so they didn't form vertical angles.
Teacher: What intersecting roads did you measure?
Student: I measured the angles of the intersection near my house. That's where Diagonal Road and 10th Ave meet.
Teacher: Let's all find that intersection on our maps so we can have a closer look. It's right near the Post Office and Hospital. Was everyone able to locate the intersection of Diagonal Road and 10th Ave? Although you didn't have vertical angles, were you able to make any other observations?
Student: I noticed that my angles added up to 180º. So did all the other angles.
Teacher: I agree that the two angles at the intersection where you live add to 180º, but what do you mean when you say, "So did all the other angles?"
Student: I mean that if you add up the angles that are next to each other, you get 180º.
Teacher: Did others find that to be true?
Student: I don't know. I need a minute to look at my drawings, because I didn't notice that. I think you might be right.
Student: My drawings show that neighboring angles add up to 180º.
Teacher: What is the name we give to neighboring angles, or angles that are next to each other?
Student: Aren't they called adjacent angles?
Teacher: Yes, adjacent angles are neighbors. They share a common side and a common vertex. If these angles add to 180º, what does that angle look like?
Student: A straight line.
Teacher: Straight angles measure 180º. And what do we call angles that add to 180º?
Student: Either supplementary or complementary, but I never remember which is which.
Teacher: Here's an idea to help you remember. Try using alliteration. Supplementary - straight, as in a straight angle; complementary - corner, as in a right angle. Now I'll ask the question again. What do we call angles that add to 180º?
Student: That's easy. They're supplementary, because they form a straight angle.
Teacher: Great! I think we're on to something. Last question for today. Are all adjacent angles either complementary or supplementary? Talk with your partner and be prepared to justify your answer.
Resources
Teacher Notes
- Students will benefit from multiple opportunities to draw and measure angles. This allows the teacher to discuss angles as movement (rotation), geometric shapes, space between intersecting lines, and measures.
- Many students may be familiar with the use of the notation ∠ A when referring to angle A. However, most will be unfamiliar with the use of m∠ A = 30o to denote the measure of angle A as 30o and will need explicit instruction.
- Students have had limited encounters with angles that require three points for naming. It is important to point out that two lines intersecting at Point A form four angles. Therefore, referring to "angle A" is ambiguous, and it is necessary to name each angle using three points. Students must also learn that when using three points to name angles, the vertex must be listed in the middle. For example, the vertex of ∠ ADF is point D. This angle can also be written as ∠ FDA.
- When students mix up the terms supplementary and complementary, remind them of the definitions and use the alliteration that complementary angles are corners and supplementary angles are straight.
- Students may believe that complementary and supplementary angles must be adjacent to (touching) each other because this is how they are often drawn. Draw examples of complementary and supplementary angles that are not adjacent, or whose orientation does not suggest that their sum is 90o or 180o. Also, provide examples of angles, both adjacent and nonadjacent, which are neither complementary or supplementary.
- The length of the rays shown in a drawing have no bearing on the measure of an angle. Angles are congruent because they have the same measure. Sharing an example like the one shown below may be helpful to demonstrate this.
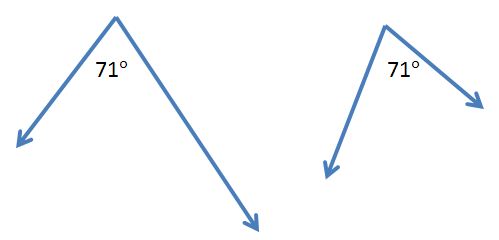
- Point out that intersecting lines creating vertical angles form an "X," and that vertical angles do not share sides.
- The definition of vertical angles is based on the position of the angles. The definitions of complementary and supplementary angles is based on the measures of the angles and not on the position of the angles.
- Providing experiences in determining and using benchmark angles will help students correctly read a protractor. Estimating that an angle is less than 90º should prevent a student from misreading a measurement of 150º for a 30º angle. Students can develop a repertoire of benchmark angles, including right angles, straight angles and 45º angles. They should be able to offer reasonable estimates for the measurement of any angle between 0º and 180º. Checking the reasonableness of a measurement should be a part of the process.
Interactive Supplementary Angles
This site allows students to click and drag around points to discover the relationship of supplementary angles.
This site allows students to click and drag around points to discover the relationship of vertical angles.
Exploring Special Pairs of Angles
Here, students can try an activity using Geometer's Sketchpad.
Wyatt, K., Lawrence, A. and Foletta, G. (2004). Geometry Activities for Middle School Students. (pp. 40-41). Emeryville, CA: Key Curriculum Press.
New Vocabulary
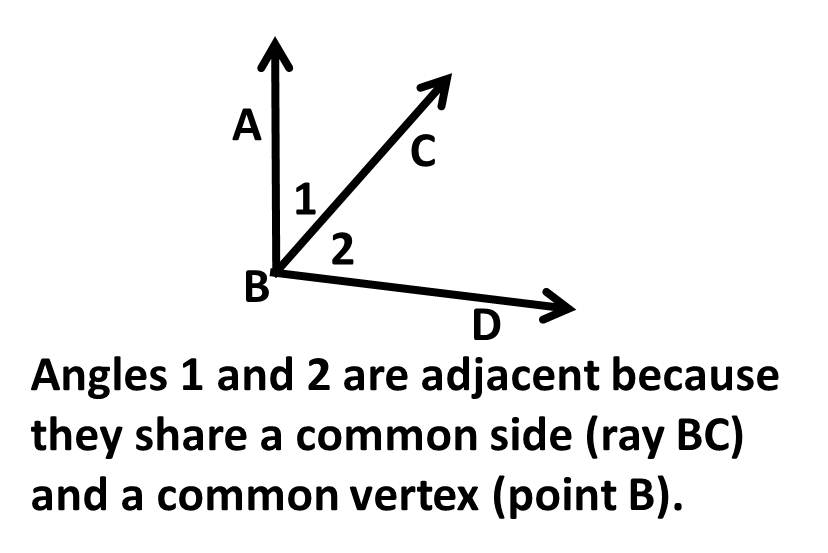
adjacent angles: two angles that have a common side and common vertex.
Example:
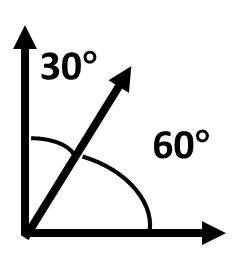
complementary angles: two angles whose measures add to 90º.
Example: 30º and 60º are complementary angles.
exterior angle: in a triangle, the angle between one side and the extension of an adjacent side.
Example:

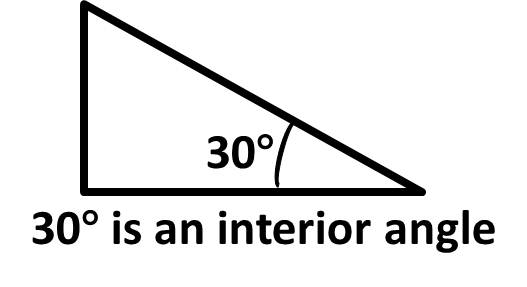
interior angle: an angle inside a shape.
Example:
intersecting lines: lines that cross or meet.
Example:

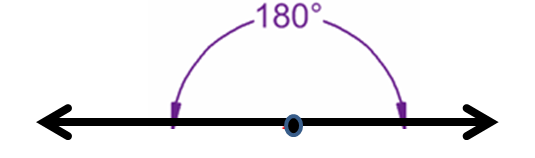
straight angle: an angle measuring 180º.
Example:
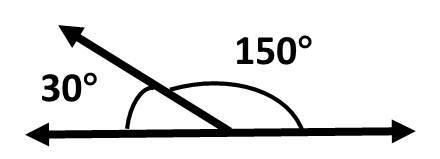
supplementary angles: two angles whose measures add to 180º.
Example: 30º and 150º are supplementary angles
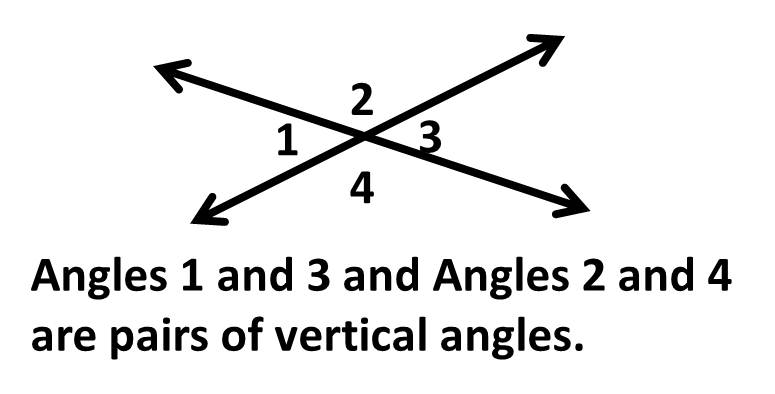
vertical angles: opposite angles formed by the intersection of two lines. Vertical angles have equal measures.
Example:
Reflection - Critical questions regarding the teaching and learning of these benchmarks
- How were concepts introduced through problem-solving or reasoning experiences?
- How did instruction and student tasks further develop students' understanding of cause and effect relationships in geometric figures?
- What strategies and accommodations were used so that all students experienced a common foundation?
- What is the evidence that students are making conjectures, explaining their ideas and questioning solutions that do not make sense to them?
Materials
- Kilpatrick, J., Martin, W., & Schifter, D. (Eds.). (2003). A Research Companion to Principles and Standards for School Mathematics. Chapter 12, Research on Students' Understanding of Angle Measure. ( p. 187). Reston, VA: National Council of Teachers of Mathematics, Inc.
- Keeley, P., & Rose, C. (2006). Mathematics Curriculum Topic Study. Thousand Oaks, CA: Corwin Press.
- Kilpatrick, J., Martin, W., & Schifter, D. (Eds.). (2003). A Research Companion to Principles and Standards for School Mathematics. Reston, VA: National Council of Teachers of Mathematics, Inc.
- Minnesota's K-12 Mathematics Frameworks. (1998). St. Paul, MN: SciMathMN.
- National Council of Teachers of Mathematics. (2000). Principles and Standards for School Mathematics. Reston, VA: National Council of Teachers of Mathematics, Inc.
- Wyatt, K., Lawrence, A., & Foletta, G. (2004). Geometry Activities for Middle School Students. Emeryville, CA: Key Curriculum Press.
Assessment
(DOK: Level 1)
1. Find m∠4 in the rectangle below.
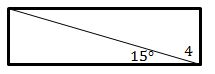
Answer: 75°
(DOK :Level 1)
2. Which angle is supplementary to 42°?
a. 42° b. 48° c. 90° d. 138°
Answer: d
(DOK: Level 2)
3. Find m∠A in the drawing below.
a. 20° b. 26° c. 46° d. 134°
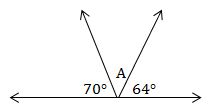
Answer: c
(DOK :Level 2)
4. Use the X below to answer the following questions.

m∠1 = ____________
m∠2 = ____________
m∠3 = ____________
Answers: m∠1= 60o ; m∠2 = 120o ; m∠3 = 60o
(DOK: Level 3)
5. Find the missing angle measure. Justify your answer.
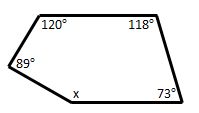
Answer: x = 140° The sum of the interior angles of a pentagon is 180(5-2) or 540.
120 + 118 + 89 + 73 + 140 = 540.
(DOK: Level 4)
6. The measures of two complementary angles have a ratio of 3:2. What is the measure of the larger angle?
Differentiation
Struggling Learners
- Check to see that students have basic understanding of vocabulary related to angles, such as vertex, rays and degrees.
- Review the symbols used to notate angles and their measures. For example, m∠ABC = 45o.
- Ask students to predict angle measures before using protractors for actual measurement to prevent misreading the protractor.
- Use graphic organizers, such as the Frayer model shown below, to support vocabulary development.
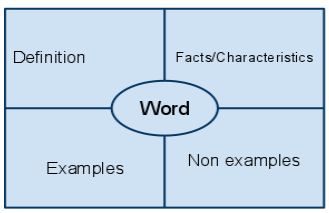
- Provide drawings and ask students to identify adjacent, complementary, right, straight, supplementary and vertical angles.
- Have students look around the room and identify angles that appear to be adjacent, complementary, right, straight, supplementary or vertical angles.
This site provides an interactive protractor to help students practice measuring angles.
This interactive site provides practice in determining missing angles of complementary angles.
- Explain that the term right angle does not come from the orientation of the angle opening to the right. Students might think that if the angle opens to the left, it is called a left angle. Point out that any angle that measures 90o is a right angle.
- Post and label pictures of acute, right, obtuse and straight angles.
- Post drawings and label adjacent, complementary, exterior, interior, right, straight, supplementary and vertical angles;
- Use graphic organizers, such as the Frayer model shown below, for vocabulary development.
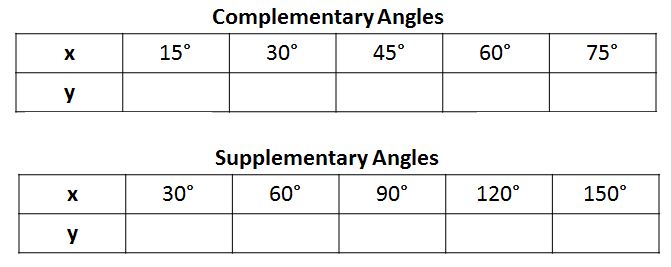
Have students find pairs of complementary or supplementary angles and use them as ordered pairs for graphing on a coordinate grid to observe linear relationships. Ask students to identify the domain (set of x-values) and range (set of y-values) and write an equation for y as a function of x for each relationship. See suggested angles below.
Parents/Admin
Administrative/Peer Classroom Observation
|
Students are: (descriptive list) |
Teachers are: (descriptive list) |
|
having multiple opportunities to draw and measure angles. |
providing hands-on experiences for students to explore angle relationships formed by intersecting lines. |
|
investigating cause and effect relationships of angles formed by intersecting lines. |
including real-world situations as a source of opportunities for exploring cause and effect relationships of angles formed by intersecting lines. |
|
using data collected during measurement investigations to observe patterns and discover angle relationships. |
posing questions that require students to make observations and draw conclusions about angle relationships based on evidence. |
|
discussing and writing about cause and effect relationships of angles formed by intersecting lines using precise mathematical language. |
providing opportunities for students to communicate their ideas. |
|
using variables to represent unknowns when solving problems involving angle relationships. |
modeling the use of algebra to solve problems involving angle relationships. |
Parent Resources
This instructional video about angles includes an introduction to supplementary and complementary angles.
Angles Part 2 - Complementary, Supplementary & Opposite Angles
This instructional video uses angle relationships of intersecting lines to solve problems.
This site provides support in understanding vertical angles and their relationship.
This site provides support in identifying adjacent angles.
This site provides support in understanding supplementary angles.
This site provides support in understanding complementary angles.
