4.3.1 Shapes
Describe, classify and sketch triangles, including equilateral, right, obtuse and acute triangles. Recognize triangles in various contexts.
Describe, classify and draw quadrilaterals, including squares, rectangles, trapezoids, rhombuses, parallelograms and kites. Recognize quadrilaterals in various contexts.
Overview
Fourth graders focus on triangles and quadrilaterals as they analyze and categorize shapes. They examine the characteristics of sides and measures of angles that make each polygon unique. They identify the specific type of triangle and quadrilateral based on their characteristics.
Fourth graders recognize, describe, classify and sketch triangles, including equilateral, right, obtuse and acute triangles. They describe, classify and draw quadrilaterals, including squares, rectangles, trapezoids, rhombuses, parallelograms and kites.
All Standard Benchmarks
4.3.1.1 Describe, classify and sketch triangles, including equilateral, right, obtuse and acute triangles. Recognize triangles in various contexts.
4.3.1.2 Describe, classify and draw quadrilaterals, including squares, rectangles, trapezoids, rhombuses, parallelograms and kites. Recognize quadrilaterals in various contexts.
4.3.1.1
Describe, classify and sketch triangles, including equilateral, right, obtuse and acute triangles. Recognize triangles in various contexts.
4.3.1.2
Describe, classify and draw quadrilaterals, including squares, rectangles, trapezoids, rhombuses, parallelograms and kites. Recognize quadrilaterals in various contexts.
What students should know and be able to do [at a mastery level] related to these benchmarks:
- identify angles as right, obtuse or acute
For example: Find 3 things in the classroom that have angles that are right (measure 90 degrees). Find 3 things that have acute angles (those that measure less than 90 degrees).
- name and classify triangles based on angle measure
- classify and accurately label quadrilaterals based on attributes
- draw, build or sketch a triangle or quadrilateral based on given criteria
For example: Sketch a triangle with 3 acute angles; or draw a quadrilateral with exactly one set of parallel sides.
- recognize triangles and quadrilaterals in everyday objects, architecture and art
Work from previous grades that supports this new learning includes:
- recognize lines (sides of shapes) as parallel, perpendicular, equilateral and congruent.
- describe and create right triangles, rectangles, parallelograms and trapezoids.
- sketch polygons based on a given number of sides and/ or vertices.
- exposure and practice with vocabulary that is essential to classifying quadrilaterals such as parallel, perpendicular and vertices.
NCTM Standards
Analyze characteristics and properties of two- and three-dimensional geometric shapes and develop mathematical arguments about geometric relationships:
Grades 3-5 Expectations:
- identify, compare, and analyze attributes of two- and three-dimensional shapes and develop vocabulary to describe the attributes;
- classify two- and three-dimensional shapes according to their properties and develop definitions of classes of shapes such as triangles and pyramids;
- investigate, describe, and reason about the results of subdividing, combining, and transforming shapes;
- explore congruence and similarity;
- make and test conjectures about geometric properties and relationships and develop logical arguments to justify conclusions.
Common Core State Standards
Draw and identify lines and angles, and classify shapes by properties of their lines and angles.
4.G.1. Draw points, lines, line segments, rays, angles (right, acute, obtuse), and perpendicular and parallel lines. Identify these in two-dimensional figures.
4.G.2. Classify two-dimensional figures based on the presence or absence of parallel or perpendicular lines, or the presence or absence of angles of a specified size. Recognize right triangles as a category, and identify right triangles.
4.G.3. Recognize a line of symmetry for a two-dimensional figure as a line across the figure such that the figure can be folded along the line into matching parts. Identify line-symmetric figures and draw lines of symmetry.
Misconceptions
Students may think...
- all shapes have only one name. They may also have incorrect names for shapes.
The vocabulary or terms that are used among the general public for shapes has caused a number of problems for students learning accurate mathematical terms for polygons.
For example: diamond has been used instead of rhombus. Also "kites" used for flying are not necessarily kite shaped.
- 2-dimensional terms can be used for 3-dimensional objects.
For example: calling a sphere (ball) or cylinder (can) a circle, or referring to a rectangular prism (a door) a rectangle.
- regular polygons are the "real" shapes.
Regular polygons in posters, picture books, and in primary math books leads children to believe that the only "real" triangle is an equilateral triangle, sitting on one of its' sides. Children need exposure to many different models of commonly named shapes.
Rarely do you see a trapezoid that does not look like this (insert trapezoid that looks like face of red pattern block) or a non-equilateral triangle.
It is extremely useful to have students redefine terms, create and keep a "math Overuse glossary" of their own in the math journal or notebook, complete with sketches and labels.
- all shapes with three sides are simply called triangles.
Something new to students is learning to name a triangle based on the measure of its angle.
For example, a triangle with an obtuse angle can be labeled as such, an obtuse triangle.
- each four sided shape can be classified only one way based on its attributes.
This is very confusing since are the many different categories polygons with four sides can fit.
For example: A square fits many of the "rules" when it comes to classifying quadrilaterals. A square is a special rectangle - it has all the properties of rectangles, with the additional property that all sides must have equal length. It is also a special rhombus- it has all the properties of a rhombus with the additional property of right angles.
Vignette
Sorting Quadrilaterals
Students in this fourth grade classroom have been describing and defining quadrilaterals based on their attributes. In this lesson, they are working with a set of 15 quadrilaterals. The lesson opens with the teacher leading a discussion around organizing the quadrilaterals and ultimately placing them into a tree map.
Teacher: We have spent that past week really getting to know some specific names for quadrilaterals. We have shown them on paper, with our building kits (straws and pipe cleaners), and we have played some guessing and sorting shape games. Today, I would like you to consider a way to organize the shapes in a way that shows relationships between and among quadrilaterals. I’m thinking of a tree map that could be added to our math board. (draws a visual on board).
What questions do you have?
Throughout the next portion several different students raise their hands and are called on to give ideas and ask questions. Each is just referred to as “student” to ease reading the vignette.
Student: How do we organize it?
Teacher: I’m not sure- what do you think
Student: We could put labels for the different categories and then put the pictures below.
Student: But I think we should put the attributes under the category so we can remember and check to see if the shapes fit.
Teacher: What would that look like?
Student: Well, we could write the name of the quadrilateral on the line and the attributes below and then the pictures below that.
Student: Oh, I think I get it.
Teacher: Why don’t you get into your math teams and try it yourselves? The guidelines are:
1. You must have a place for each of the quadrilaterals we have studied.
2. You must accurately place a copy or sketch of the quadrilaterals in the category or categories where it fits.
3. You must find a way to represent a quadrilateral that fits in more than one group.
Teacher: Any questions?
Student: Can we use the quadrilaterals on the sheet and also draw some ourselves?
Teacher: Yes.
Student: Can we change how the tree map looks?
Teacher: Say more about that.
Student: Can the “branches” look different than on other maps we made- like maybe more than one branch coming from a label?
Teacher: Yes. Anything else? (pause-waits…) Okay, materials are on the round table, I’ll be around to check your progress.
Students head off in their pre-assigned groups (also known as their “math team”)-some groups have 3 members others have 4. *For the geometry unit the “math teams” assigned are mixed ability groups. There are 8 groups total. 3 different groups are documented below. The other groups are doing similar work.
Group A starts by talking strategy.
Student 1A: I think we should write the categories on small pieces of paper and then place them on the tree map that way if we want to move them we can.
Student 2A: Good idea, then we can put the pictures in the area we think they fit.
Student 3A: But what about the shapes that are lots of things, like the square is a rectangle and a rhombus?
Student 2A: Can we make a copy of the polygons- like trace them or something?
Student 1A: She said we can use the copy she gave us and sketch our own, so we could trace them if we want them exactly the same or we could try to make our own.
They start dividing up tasks and getting their materials organized.
Group B is busy working but not much discussion yet. Two of them are cutting out the polygons, another is placing names of quadrilaterals onto the tree map on the construction paper, and the last group member is talking.
Student 1B: What should I do? Should I draw some more shapes? What do you think we need? I like making parallelograms. Do we need those?
Student 2B: (cutting pre-made shapes from paper) I only see one kite, it might be good to have another one. And maybe more squares- we need one for each category.
Student 1B: I wonder if I can use the dot or square paper to cut squares, those are tricky (walks off to get different paper).
Student 3B: How many categories do we need? I have parallelogram, rhombus, kite, and square- oh, I’ll look in my notebook-
Student 4B: You missed rectangle and trapezoid and I think you spelled some of those words wrong.
Group C has all their materials in front of them. They send one member off to see what Group A is doing and another member goes to see what Group B is doing.
The group members all return:
Student 1C: Group A is making moveable labels until they figure out what to do.
Student 2C: Group B is already writing their labels- it looks kind of messy.
You can see what categories they all fit in...
Student 2C: Yes! Work backward- I love working backward!
Student 4C: And then we can do the kind of map I asked about, where one shape might have branches from lots of categories coming to it or something like that!
Student 2C: Another great idea- we are going to rock this!
They get started cutting out shapes while Student 4C starts sketching out ideas for a possible map.
Groups continue working for approximately thirty minutes. Groups are then asked to present the final map and summarize their work.
Note: Entire group goes up to present. Each group chooses one person to present final work but all group members answer audience questions.
All groups present but for the purpose of this vignette, only A, B, and C are represented.
The teacher decided to start with Group B because they sorted but did not recognize the relationships between and among shapes.
Group B presentation:
Group B work
Student 1B: We started by dividing up jobs, writing, cutting and then making extra shapes. We just labeled the paper with each category and cut out or made a shape for each category. We didn’t put the definitions for them -we forgot- and we didn’t put the square in a different category either. But, I still think we did it right. We have all the labels and we have a shape in each one. Questions?
Student: How did you decide to do it that way?
Student 2B: (Shrugs) I don’t know, I just started cutting out shapes and someone else started writing, we didn’t really decide or make a plan really- but we all agreed with what we were doing.
Teacher: Everyone look at Group B’s work. Do all of the shapes fit in the categories where they placed them?
Student: Well....they all fit but some of them could fit in other parts. It looks like there is a rectangle under the Parallelogram part. I think it should be moved with the other rectangles.
Student: But wait....it could go in either place because a rectangle is also a parallelogram.
Actually, you could move all the rectangles under the parallelogram. The squares are already there and they are rectangles.
Teacher: I hear you saying that some quadrilaterals belong in more than one group. Let’s see how another group thought about shapes that belong in more than one group.
Group A presentation:
Group A work
Student 1A: We did a similar strategy to group B. We thought first and glued last but we started by making labels of the categories and the writing attributes of each group. Next, we found examples of quadrilaterals that fit the category- notice how we put square between rectangle and rhombus? We did this because then we could have the square pasted halfway into both categories.
Teacher: What do you think? Do all of the shapes fit in the categories where they placed them?
Student: I don’t see any that are out of place. Other students nod in agreement.
Teacher: What relationships between these shapes is Group A trying to show?
Student: They are showing that squares are rectangles and rhombuses because they put them between but I wouldn’t have known that if they hadn’t told us.
Teacher: Do you agree that all squares are rectangles and that all squares are rhombuses? Why or Why not? Talk to the person sitting next to you and be ready to share with the class.
It is important that every student is able to articulate this understanding. The teacher has been listening to student conversations and selects a student to share his thinking.
Student: All squares are rectangles because they have four right angles and opposite sides are parallel.
Teacher: Yes, all squares are rectangles because they have four right angles and opposite sides are parallel. The teacher records on chart paper and asks students to read it aloud. This will be posted on the math wall as a class reference.
Student: All squares are rhombuses because they have four sides that are the same length.
Teacher: Yes, all squares are rhombuses because they have four sides that are the same length. The teacher records on chart paper and asks students to read it aloud. This will be posted on the math wall as a class reference.
Teacher: What does Group A’s work tell us about parallelograms?
Student: We know that all rectangles are parallelograms and all squares are parallelograms and all rhombuses are parallelograms because the arrows point that way. The arrows all go to the parallelogram.
Teacher: Correct. Why are squares, rectangles and rhombuses all parallelograms? Talk to the person sitting next to you. Be ready to share your thinking.
The teacher asks students to share their thinking and records it for the math wall.
Student 2A: Oh.... we should have put an arrow from square to rhombus and from square to rectangle just like we did for the parallelograms instead of just a line connecting them.
Teacher: Let’s see how another group thought about how to show these relationships.
Summary of Group C presentation:
Group C work
Student 4C: We started by cutting out quadrilaterals and thinking about where they belonged. We got stuck for a while because we knew all the categories that square fit into so we started there- we worked backward really. We finally realized that the rectangle, rhombus and square were all parallelograms and that made our map more interesting. So what we did next was we made a tree map coming from the parallelogram. We ended up with three main categories, parallelogram, trapezoid and kite. Then we put an example in for each quadrilateral and color coded them.
See how the square is traced in three colors because it fit all three criteria? That is basically what we did. Questions?
Student: Why isn’t your rectangle traced in green? That is the one that says 2 sets of parallel sides.
Student 1C: Oh! I was in charge of that and I just forgot! I never noticed! It should be green too!
Student: I really like the way you did that map. It is easy for me to see how the shapes go together.
Teacher: Using what you know about shapes and what we just added to our math wall, are the shapes placed in appropriate categories? How do you know? Talk to the person sitting next to you and be ready to share your thinking.
The students agreed with the placement and could, for the most part, explain the relationships shown in Group C’s work.
These fourth graders used what they knew about the characteristics of shapes to create and organize a diagram. Seeing a model of the relationships that exist between and among shapes helps students solidify this understanding. Some representations were more complex but all demonstrated a level of student understanding. The teacher was able to use the students work to build understanding while clarifying relationships between and among shapes.
Resources
- Students may need support in further development of previously studied concepts and skills.
- The use of computer drawing programs will enable students to "draw" shapes using precise lines and angles.
- Mathematics tools such as geoboards, geoboard dot paper, pattern blocks and triangle block paper can aid students in building and recording the different polygons students are studying.
- Teaching all the attributes of all the different quadrilaterals can overwhelm students. Focusing on 2 or 3 shapes will help students make meaning and make connections between shapes and their attributes.
- Using a math notebook is a great way to keep notes and even a glossary to record new learning and vocabulary. It can serve as a place for students to record their thinking as they sketch, label and categorize different polygons.
- Dynamic Paper
Need a set of pattern blocks where all shapes have one-inch sides? You can create these and more with the Dynamic Paper tool. Place the images you want, then export it as a PDF or as a JPEG image for use in other applications.
adapted from: Van de Walle J. (2006) and Van de Walle, J., & Lovin, L. (2006).
The van Hiele Levels of Geometric Thought is a hierarchy describing the way that students learn to reason about shapes and other geometric ideas. There are five levels in the hierarchy that are deemed to be sequential. Students need to move through each prior level before moving on to the next. Student thinking about particular concepts will likely be at different levels at any given time. The levels describe how we think and what types of geometric ideas we think about, rather than how much knowledge we have. Movement through the levels depends on the types and amount of experiences students have with geometry. Instruction that takes place at a level higher than the students' functional level will be ineffective. Many adults remain at level 1 even though they have had a geometry course in high school. With appropriate experiences, however, students can reach level 2 in elementary school.
Level 0 - Visualization
The objects of thought at level 0 are shapes and what they "look like."
Students may be able to talk about the properties of the shapes, but the properties are not thought about explicitly. They characterize individual shapes based on appearance. "It is a square because it looks like a square." At this level, students think shapes "change" or have different properties when rotated or rearranged.
The products of thought at level 0 are classes or groupings of shapes that seem to be alike.
Level 1 - Analysis
The objects of thought at level 1 are classes of shapes rather than individual shapes.
Students are able to think of properties (number of sides, angles, parallel sides, etc.) of a shape rather than focusing on the appearance of a shape. A student operating at this level might list all the properties the student knows about a shape, but not discern which properties are necessary and which are sufficient to identify the shape. "It is a square because it has square corners and the sides are the same." Though students see properties of shapes, they cannot make generalizations about how different shapes relate to one another. Students at this level will not see the relationship of a square to a rectangle.
The products of thought at level 1 are the properties of shapes.
Level 2 - Informal Deduction or Abstraction
The objects of thought at level 2 are the properties of shapes.
Students develop relationships between and among properties. Shapes can be
classified using minimal characteristics. "Rectangles are parallelograms with a
right angle." Students at level 2 will be able to follow an informal deductive argument about shapes and their properties. While many adults remain at level 0 or level 1, with appropriate experiences, most students could reach level 2 by the end the elementary grades.
The products of thought at level 2 are relationships among properties of geometric objects.
Level 3 - Deduction
The objects of thought at level 3 are relationships among properties of geometric
objects.
Students work with abstract statements about geometric properties and make
conclusions based on logic. This is the level of a traditional high school geometry
course.
The products of thought at level 3 are deductive axiomatic systems for geometry.
Level 4 - Rigor
The objects of thought at level 4 are deductive axiomatic systems for geometry.
Students operating at this level focus on axiomatic systems, not just deductions
within the system. This is usually at a level of a college geometry course.
The products of thought at level 4 are comparisons and contrasts among different
axiomatic systems of geometry.
Note: In some literature the van Hiele Levels of Geometric Thought are
labeled 1-5 rather than 0-4.
- Good questions, and good listening, will help children make sense of the mathematics, build self-confidence and encourage mathematical thinking and communication. A good question opens up a problem and supports different ways of thinking about it. The best questions are those that cannot be answered with a "yes" or a "no."
Getting Started
What do you need to find out?
What do you know now? How can you get the information? Where can you begin?
What terms do you understand/not understand?
What similar problems have you solved that would help?
While Working
How can you organize the information?
Can you make a drawing (model) to explain your thinking? What are other possibilities?
What would happen if...?
Can you describe an approach (strategy) you can use to solve this?
What do you need to do next?
Do you see any patterns or relationships that will help you solve this?
How does this relate to...?
Why did you...?
What assumptions are you making?
Reflecting about the Solution
How do you know your solution (conclusion) is reasonable? How did you arrive at your answer?
How can you convince me your answer makes sense?
What did you try that did not work? Has the question been answered?
Can the explanation be made clearer?
Responding (helps clarify and extend their thinking)
Tell me more.
Can you explain it in a different way?
Is there another possibility or strategy that would work?
Is there a more efficient strategy?
Help me understand this part ...
(Adapted from They're Counting on Us, California Mathematics Council, 1995)
NCTM Illuminations
Learning Objective: Students will identify, compare, and analyze attributes of rectangles and parallelograms through physical and mental manipulation.
Learning Objective: Students will
identify and describe characteristics of geometric figures
compare the properties of geometric figures
understand terminology related to geometric figures
Additional Instructional Resources
Gavin, M., Belkin, L., Soinelli, A., & St. Marie, J. (2001). Navigating through geometry in grades 3-5. Reston, VA: National Council of Teachers of Mathematics.
Small, M. (2009). Good questions: great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Van de Walle, J., Karp, K., Bay-Williams, J. (2010). Elementary and middle school mathematics: teaching developmentally. (7th ed.) Boston, MA: Allyn & Bacon.
Van de Walle, J. & Lovin, L. (2006). Teaching student-centered mathematics grades 3-5. Boston, MA: Pearson Education.
acute angle: an angle that measures more than 0° and less than 90°
acute triangle: a triangle with all three angles measuring less than 90°
obtuse angle: an angle that measures more than 90° and less than 180°
obtuse triangle: a triangle with one angle that measures more than 90°
right angle: an angle that measures exactly 90°
right triangle: a triangle with an angle that measures exactly 90°
equilateral triangle: a triangle whose 3 sides have the same length and whose 3 angles have the same measure (60 degrees)
kite: a quadrilateral that has two pairs of consecutive congruent sides, but in which opposite sides are not congruent
quadrilateral: a polygon with four sides
rhombus: a parallelogram with all sides of equal length
trapezoid: a quadrilateral with exactly one pair of parallel sides
"Vocabulary literally is the
key tool for thinking."
Ruby Payne
Mathematics vocabulary words describe mathematical relationships and concepts and cannot be understood by simply practicing definitions. Students need to have experiences communicating ideas using these words to explain, support, and justify their thinking.
Learning vocabulary in the mathematics classroom is contingent upon the following:
Integration: Connecting new vocabulary to prior knowledge and previously learned vocabulary. The brain seeks connections and ways to make meaning which occurs when accessing prior knowledge.
Repetition: Using the word or concept many times during the learning process and connecting the word or concept with its meaning. The role of the teacher is to provide experiences that will guarantee connections are made between mathematical concepts, relationships, and corresponding vocabulary words.
Meaningful Use: Multiple and varied opportunities to use the words in context. These opportunities occur when students explain their thinking, ask clarifying questions, write about mathematics, and think aloud when solving problems. Teachers should be constantly probing student thinking in order to determine if students are connecting mathematics concepts and relationships with appropriate mathematics vocabulary.
Strategies for vocabulary development
Students do not learn vocabulary words by memorizing and practicing definitions. The following strategies keep vocabulary visible and accessible during instruction.
Mathematics Word Bank: Each unit of study should have word banks visible during instruction. Words and corresponding definitions are added to the word bank as the need arises. Students refer to word banks when communicating mathematical ideas which leads to greater understanding and application of words in context.
Labeled pictures and charts: Diagrams that are labeled provide opportunities for students to anchor their thinking as they develop conceptual understanding and increase opportunities for student learning.
Frayer Model: The Frayer Model connects words, definitions, examples and non-examples.
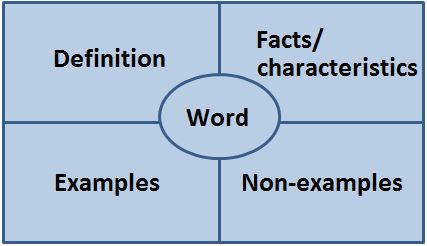
Example/Non-example Charts: This graphic organizer allows students to reason about mathematical relationships as they develop conceptual understanding of mathematics vocabulary words. Teachers should use these during the instructional process to engage student in thinking about the meaning of words.

Vocabulary Strips: Vocabulary strips give students a way to organize critical information about mathematics vocabulary words.
|
word |
definition |
illustration |
Encouraging students to verbalize thinking by drawing, talking, and writing, increases opportunities to use the mathematics vocabulary words in context.
Additional Resources for Vocabulary Development
Murray, M. (2004). Teaching mathematics vocabulary in context. Portsmouth, NH: Heinemann.
Sammons, L. (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
Reflection - Critical questions regarding the teaching and learning of these benchmarks.
What are the key ideas related to triangles and rectangles at the fourth grade level? How do student misconceptions interfere with mastery of these ideas?
What experiences do students need in order to develop an understanding of the classification of triangles and rectangles ?
What relationships should fourth graders make between triangles? rectangles?
How will you know fourth graders have made these connections?
When checking for student understanding of triangles or rectangles, what should teachers
- listen for in student conversations?
- look for in student work?
- ask during classroom discussions?
Examine student work related to a task involving classifying triangles or rectangles. What evidence do you need to say a student is proficient? Using three pieces of student work, determine what student understanding is observed through the work.
How can teachers assess student learning related to these benchmarks?
How are these benchmarks related to other benchmarks at the fourth grade level?
Professional Learning Community Resources
Bamberger, H., Oberdorf, C., & Schultz-Ferrell, K. (2010). Math misconceptions prek-grade 5: From misunderstanding to deep understanding. Portsmouth, NH: Heinemann.
Chapin, S., and Johnson, A. (2006). Math matters: Understanding the math you teach, grades K-8. (2nd ed.). Sausalito, CA: Math Solutions Press.
Chapin, S., O'Connor, C., & Canavan Anderson, N. (2009). Classroom discussions: Using math talk to help students learn (Grades K-6). Sausalito, CA: Math Solutions.
Fosnot, C., & Dolk, M. (2002). Young mathematicians at work: Multiplication and division. Portsmouth, NH: Heinemann.
Hyde, Arthur. (2006). Comprehending math adapting reading strategies to teach mathematics, K-6. Portsmouth, NH: Heinemann.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for elementary school teachers. Reston, VA: National Council of Teachers of Mathematics.
Otto, A., Caldwell, J., Wallus Hancock, S., & Zbiek, R.(2011). Developing essential understanding of multiplication and division for teaching mathematics in grades 3 - 5. Reston, VA.: National Council of Teachers of Mathematics.
Parrish, S. (2010). Number talks: Helping children build mental math and computation strategies grades K-5. Sausalito. CA: Math Solutions.
Sammons, L., (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
Schielack, J. (2009). Focus in grade 3, teaching with curriculum focal points. Reston, VA: National Council of Teachers of Mathematics.
Bamberger, H., Oberdorf, C., & Schultz-Ferrell, K. (2010). Math misconceptions prek-grade 5: From misunderstanding to deep understanding. Portsmouth, NH: Heinemann.
Bender, W. (2009). Differentiating math instruction: Strategies that work for k-8 classrooms! Thousand Oaks, CA: Corwin Press.
Bresser, R., Melanese, K., & Sphar, C. (2008). Supporting English language learners in math class, grades k-2. Sausalito, CA: Math Solutions Publications.
Burns, Marilyn. (2007). About teaching mathematics: A k-8 resource (3rd ed.). Sausalito, CA: Math Solutions Publications.
Burns, M. (Ed). (1998). Leading the way: Principals and superintendents look at math instruction. Sausalito, CA: Math Solutions.
Caldera, C. (2005). Houghton Mifflin math and English language learners. Boston, MA: Houghton Mifflin Company.
Carpenter, T., Fennema, E., Franke, M., Levi, L., & Empson, S. (1999). Children's mathematics cognitively guided instruction. Portsmouth, NH: Heinemann.
Cavanagh, M. (2006). Math to learn: A mathematics handbook. Wilmington, MA: Great Source Education Group, Inc.
Chapin, S., & Johnson, A. (2006). Math matters: Understanding the math you teach, grades K-8. (2nd ed.). Sausalito, CA: Math Solutions Press.
Chapin, S., O'Connor, C., & Canavan Anderson, N. (2009). Classroom discussions: Using math talk to help students learn (Grades K-6). Sausalito, CA: Math Solutions.
Dacey, L., & Salemi, R. (2007). Math for all: Differentiating instruction k-2. Sausalito, CA: Math Solutions.
Donovan, S., & Bradford, J. (Eds). (2005). How students learn: Mathematics in the classroom. Washington, DC: National Academies Press.
Dougherty, B., Flores, A., Louis, E., & Sophian, C. (2010). Developing essential understanding of number & numeration pre-k-grade 2. Reston, VA: National Council of Teachers of Mathematics.
Felux, C., & Snowdy, P. (Eds.). ( 2006). The math coach field guide: Charting your course. Sausalito, CA: Math Solutions.
Fuson, K., Clements, D., & Beckmann, S. (2009). Focus in grade 2 teaching with curriculum focal points. Reston, VA: National Council of Teachers of Mathematics.
Hyde, Arthur. (2006). Comprehending math adapting reading strategies to teach mathematics, K-6. Portsmouth, NH: Heinemann.
Kilpatrick, J., & Swafford, J. (Eds). (2001). Adding it up: Helping children learn mathematics. Washington, DC: National Academies Press.
Leinwand, S. (2000). Sensible mathematics: A guide for school leaders. Portsmouth, NH: Heinemann.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for elementary school teachers. Reston, VA: National Council of Teachers of Mathematics.
Murray, M. (2004). Teaching mathematics vocabulary in context. Portsmouth, NH: Heinemann.
Murray, M., & Jorgensen, J. (2007). The differentiated math classroom: A guide for teachers k-8. Portsmouth, NH: Heinemann.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: NCTM.
Parrish, S. (2010). Number talks: Helping children build mental math and computation strategies grades K-5. Sausalito. CA: Math Solutions.
Reeves, D. (2007). Ahead of the curve: The power of assessment to transform teaching and learning. Indiana: Solution Tree Press.
Sammons, L. (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
Schielack, J., Charles, R., Clements, D., Duckett, P., Fennell, F., Lewandowski, S., ... & Zbiek, R. M. (2006). Curriculum focal points for prekindergarten through grade 8 mathematics: A quest for coherence. Reston, VA: NCTM.
Seeley, C. (2009). Faster isn't smarter: Messages about math teaching and learning in the 21st century. Sausalito, CA: Math Solutions.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Van de Walle, J., Karp, K., Bay-Williams, J. (2010). Elementary and middle school mathematics: Teaching developmentally. (7th ed.). Boston, MA: Allyn & Bacon.
Van de Walle, J. A., & Lovin, L. H. (2006). Teaching student-centered mathematics grades K-3. Boston, MA: Pearson Education.
West, L., & Staub, F. (2003). Content focused coaching: Transforming mathematics lessons. Portsmouth, NH: Heinemann.
Assessment
4.3.1.2
- #11 MCA III
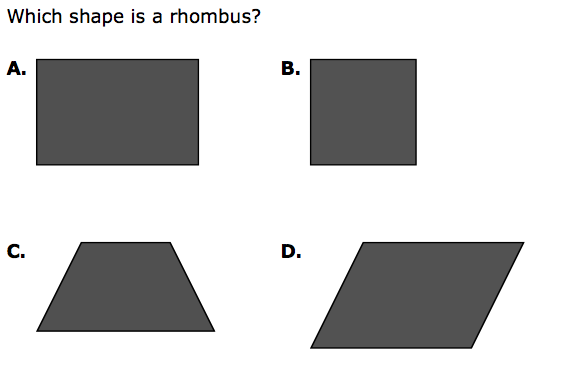
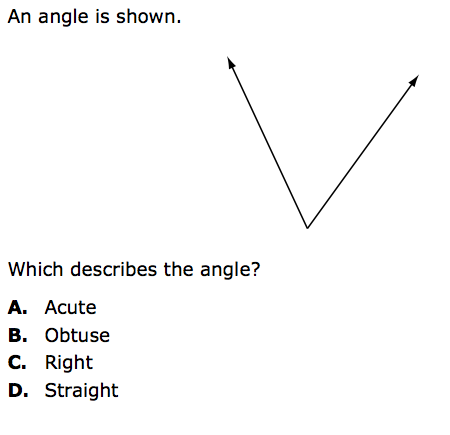
Solution:
Benchmark: 4.3.1.2
MCA III Item Sampler
Solution: A. Acute
Benchmark: 4.3.1.1
MCA III Item Sampler
- Which statement is true about an obtuse triangle?
A. It has 2 acute angles.
B. It has 2 obtuse angles.
C. It can be a right triangle.
D. It can be an acute triangle.
Solution: A. It has 2 acute angles.
Benchmark: 4.3.1.1
MCA III Item Sampler
- Which of the following shapes has exactly one set of parallel sides?
A. rhombus
B. trapezoid
C. rectangle
D. kite
Solution: B. trapezoid
Benchmark: 4.3.1.2
Differentiation
Geometry tends to be a topic that students generally can participate in with high levels of success. The vocabulary does get cumbersome, so providing the terms for students in a word bank and assisting with the math notebook will be essential.
Pre-teaching new terms, reviewing and practicing learned terms, and previewing lesson objectives can help students prepare to learn with their peers. Students of all abilities worked together in the vignette above and all groups were successful.
Concrete - Representational - Abstract Instructional Approach
The Concrete-Representational-Abstract Instructional Approach (CRA) is a research-based instructional strategy that has proven effective in enhancing the mathematics performance of students who struggle with mathematics.
The CRA approach is based on three stages during the learning process:
Concrete - Representational - Abstract
The Concrete Stage is the doing stage. The concrete stage is the most critical in terms of developing conceptual understanding of mathematical skills and concepts. At this stage, teachers use manipulatives to model mathematical concepts. The physical act of touching and moving manipulatives enables students to experience the mathematical concept at a concrete level. Research shows that students who use concrete materials develop more precise and comprehensive mental representations, understand and apply mathematical concepts, and are more motivated and on-task. Manipulatives must be selected based upon connections to the mathematical concept and the students' developmental level.
The Representational Stage is the drawing stage. Mathematical concepts are represented using pictures or drawings of the manipulatives previously used at the Concrete Stage. Students move to this level after they have successfully used concrete materials to demonstrate conceptual understanding and solve problems. They are moving from a concrete level of understanding toward an abstract level of understanding when drawing or using pictures to represent their thinking. Students continue exploring the mathematical concept at this level while teachers are asking questions to elicit student thinking and understanding.
The Abstract Stage is the symbolic stage. Teachers model mathematical concepts using numbers and mathematical symbols. Operation symbols are used to represent addition, subtraction, multiplication and division. Some students may not make a clean transfer to this level. They will work with some symbols and some pictures as they build abstract understanding. Moving to the abstract level too quickly causes many student errors. Practice at the abstract level will not lead to increased understanding unless students have a foundation based upon concrete and pictorial representations.

Additional Resources
Bender, W. (2009). Differentiating math instruction: Strategies that work for k-8 classrooms! Thousand Oaks, CA: Corwin Press.
Dacey, L., & Lynch, J. (2007). Math for all: Differentiating instruction grades 3-5. Sausalito, CA: Math Solutions.
Murray, M. & Jorgensen, J. (2007). The differentiated math classroom: A guide for teachers k-8. Portsmouth, NH: Heinemann.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Van de Walle, J., Karp, K., Bay-Williams, J. (2010). Elementary and middle school mathematics: Teaching developmentally. (7th ed.) Boston, MA: Allyn & Bacon.
Van de Walle, J. & Lovin, L. (2006). Teaching student-centered mathematics grades 3-5. Boston, MA: Pearson Education.
Connect characteristics of specific shapes to representations of those shapes. Clearly label characteristics to provide a reference for student use.
Many of the terms in geometry are derived from different languages. Providing all students an opportunity to do a "language study" on the origin of specific terms is not only useful to the ELL learner, it is useful to all learners.
- Word banks need to be part of the student learning environment in every mathematics unit of study. Refer to these throughout instruction.
- Word Use vocabulary graphic organizers such as the Frayer model (see below) to emphasize vocabulary words count, first, second, third, etc.

- Sentence Frames
Math sentence frames provide support that English Language Learners need in order to fully participate in math discussions. Sentence frames provide appropriate sentence structure models, increase the likelihood of responses using content vocabulary, help students to conceptualize words and build confidence in English Language Learners.
Sample sentence frames related to these benchmarks:
|
This is a ____________________ because ____________________________________. |
|
These are all ______________________ because ______________________________. |
When assessing the math skills of an ELL student it is important to determine if the student has difficulty with the math concept or with the language used to describe the concept and conceptual understanding.
Additional ELL Resources
Bresser, R., Melanese, K., & Sphar, C. (2008). Supporting English language learners in math class, grades k-2. Sausalito, CA: Math Solutions Publications.
Will any three sides, regardless of length, create a triangle? In answering this question, they will learn that side a + side b must be more than the length of side c, a + b > c. They could also work on looking at relationships between angle measure and side lengths.
Additional Resources
Bender, W. (2009). Differentiating math instruction-strategies that work for k-8 classrooms! Thousand Oaks, CA: Corwin Press.
Dacey, L., & Lynch, J. (2007). Math for all: Differentiating instruction grades 3-5. Sausalito, CA: Math Solutions.
Murray, M. & Jorgensen, J. (2007). The differentiated math classroom A guide for teachers k-8. Portsmouth, NH: Heinemann.
Small, M. (2009). Good questions: Great ways to differentiate mathematics instruction. New York, NY: Teachers College Press.
Parents/Admin
Administrative/Peer Classroom Observation
|
Students are ... |
Teachers are ... |
|
describing and naming triangles and quadrilaterals in terms of types of angles and parallel and perpendicular sides. |
providing practice in the form of polygons to label terms and definitions for students to refer to, a "word bank" that is accessible to students (either on the wall or in their notebooks or both). |
|
drawing triangles and quadrilaterals on paper, with material and in the classroom, the school and art. |
assisting students as needed, watching for accuracy, making tools and examples available to students. |
|
sorting and classifying triangles and quadrilaterals based on angle measure, side and angle number, side length and other attributes that distinguish one polygon from the other. |
offering examples to sort, providing a forum in which students can discuss/debate their reasons for placing a polygon in a certain group, or giving it a specific label guiding students toward the most specific label for each polygon. |
What should I look for in the mathematics classroom?
(Adapted from SciMathMN,1997)
What are students doing?
- Working in groups to make conjectures and solve problems.
- Solving real-world problems, not just practicing a collection of isolated skills.
- Representing mathematical ideas using concrete materials, pictures and symbols. Students know how and when to use tools such as blocks, scales, calculators, and computers.
- Communicating mathematical ideas to one another through examples, demonstrations, models, drawing, and logical arguments.
- Recognizing and connecting mathematical ideas.
- Justifying their thinking and explaining different ways to solve a problem.
What are teachers doing?
- Making student thinking the cornerstone of the learning process. This involves helping students organize, record, represent, and communicate their thinking.
- Challenging students to think deeply about problems and encouraging a variety of approaches to a solution.
- Connecting new mathematical concepts to previously learned ideas.
- Providing a safe classroom environment where ideas are freely shared, discussed and analyzed.
- Selecting appropriate activities and materials to support the learning of every student.
- Working with other teachers to make connections between disciplines to show how math is related to other subjects.
- Using assessments to uncover student thinking in order to guide instruction and assess understanding.
Additional Resources
For Mathematics Coaches
Chapin, S. and Johnson, A. (2006). Math matters: Understanding the math you teach: Grades k-8. (2nd ed.). Sausalito, CA: Math Solutions.
Donovan, S., & Bradford, J. (Eds). (2005). How students learn: Mathematics in the classroom. Washington, DC: National Academies Press.
Felux, C., & Snowdy, P. (Eds.). ( 2006). The math coach field guide: Charting your course. Sausalito, CA: Math Solutions.
Sammons, L., (2011). Building mathematical comprehension: Using literacy strategies to make meaning. Huntington Beach, CA: Shell Education.
West, L., & Staub, F. (2003). Content focused coaching: Transforming mathematics lessons. Portsmouth, NH: Heinemann.
For Administrators
Burns, M. (Ed). (1998). Leading the way: Principals and superintendents look at math instruction. Sausalito, CA: Math Solutions.
Kilpatrick, J., & Swafford, J. (Eds). (2001). Adding it up: Helping children learn mathematics. Washington, DC: National Academies Press.
Leinwand, S. (2000). Sensible mathematics: A guide for school leaders. Portsmouth, NH: Heinemann.
Lester, F. (2010). Teaching and learning mathematics: Transforming research for school administrators. Reston, VA: National Council of Teachers of Mathematics.
Seeley, C. (2009). Faster isn't smarter: Messages about math teaching and learning in the 21st century. Sausalito, CA: Math Solutions.
Parent Resources
Mathematics handbooks to be used as home references:
Cavanagh, M. (2004). Math to Know: A mathematics handbook. Wilmington, MA: Great Source Education Group, Inc.
Cavanagh, M. (2006). Math to learn: A mathematics handbook. Wilmington, MA: Great Source Education Group, Inc.
Helping your child learn mathematics
Provides activities for children in preschool through grade 5
What should I look for in the mathematics program in my child's school? A Guide for Parents developed by SciMathMN
Help Your Children Make Sense of Math
Ask the right questions
In helping children learn, one goal is to assist children in becoming critical and independent thinkers. You can help by asking questions that guide, without telling them what to do.
Good questions, and good listening, will help children make sense of the mathematics, build self-confidence and encourage mathematical thinking and communication. A good question opens up a problem and supports different ways of thinking about it. The best questions are those that cannot be answered with a "yes" or a "no."
Getting Started
What do you need to find out?
What do you know now? How can you get the information? Where can you begin?
What terms do you understand/not understand?
What similar problems have you solved that would help?
While Working
How can you organize the information?
Can you make a drawing (model) to explain your thinking? What are other possibilities?
What would happen if . . . ?
Can you describe an approach (strategy) you can use to solve this?
What do you need to do next?
Do you see any patterns or relationships that will help you solve this?
How does this relate to ...?
Can you make a prediction?
Why did you...?
What assumptions are you making?
Reflecting about the Solution
How do you know your solution (conclusion) is reasonable? How did you arrive at your answer?
How can you convince me your answer makes sense?
What did you try that did not work?
Has the question been answered?
Can the explanation be made clearer?
Responding (helps clarify and extend their thinking)
Tell me more.
Can you explain it in a different way?
Is there another possibility or strategy that would work?
Is there a more efficient strategy?
Help me understand this part...
Adapted from They're counting on us, California Mathematics Council, 1995.
- Literature connection: The Greedy Triangle, by Marilyn Burns
- At home or in the car activities:
I Spy is a great one for helping your child see polygons in contexts. Be sure to acknowledge that the door has the overall shape of a rectangle but that it is 3-dimensional. You will be surprised the places you will find shapes in your house. The ceiling of a room with the "tray" effect will have trapezoids! So will many lampshades! The folding drying rack for laundry has rhombuses!
Ten Questions is a twist on an old stand by. You or your child pick a polygon (one of the triangles or quadrilaterals stated in the standard). The person who is guessing can ask only "yes" or "no" questions and cannot guess the actual polygon until they have asked 9 of the ten questions. Question #10 is the actual guess, of course you can modify the rules if you wish. This game helps practice vocabulary and eliminates premature guessing at the shape. Here is a sample round:
Parent: I have a shape in mind.
Child: Does it have 3 angles? (1)
Parent: No
Child: Does it have at least one set of parallel sides? (2)
Parent: Yes
Child: Would it be considered a parallelogram? (3)
Parent: Yes
Child: Does it have any acute angles? (4)
Parent: No
Child: Does it have right angles? (5)
Parent: Yes
Child: Does it only have right angles? (6)
Parent: Yes
Child: Are all 4 sides of equal length? (7)
Parent: Yes
Child: Could you call this shape a rectangle? (8)
Parent: Yes
Child: Would it be considered a rhombus? (9)
Parent: Yes
Child: Is the shape a square? (10)
Parent: YES!